I show the details for a complete OFDM transmitter chain at this post, and this other post provides additional related details.
The OP has clarified in a comment that there is still confusion in how the upconversion to an RF carrier is done for this waveform.
The upconversion is no different than any other baseband modulated waveform where the baseband complex waveform is directly translated to either an IF (Intermediate Frequency) or RF (Radio Frequency) carrier. This process is mathematically described as:
$$x_{RF}(t) = \text{Re}\{x(t)e^{j\omega_c t}\} \tag{1}\label{1}$$
Where $x(t)$ is the complex baseband waveform typically given in real and imaginary components as $I(t)+jQ(t)$, and $\omega_c$ is the desired carrier frequency. We note that the frequency translation to a real carrier is done with a complex multiplication with $e^{j\omega_c t}$ which itself has real and imaginary components, so we can write this as $I_c+jQ_c$, and thus Equation \ref{1} becomes:
$$x_{RF}(t) = \text{Re}\{(I(t)+jQ(t))(I_c(t)+jQ_c(t))\} = I(t)I_c(t)-Q(t)Q_c(t) \tag{2}\label{2}$$
As an implementation block diagram as given in actual hardware, Equation \ref{2} becomes:
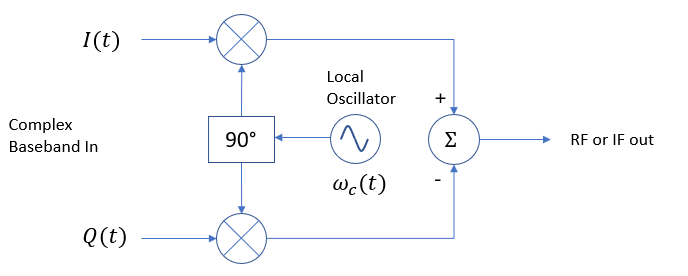
For Direct RF the output is the RF (Radio Frequency) carrier, for a Heterodyne upconverter with an intermediate IF (intermediate Frequency), the output is the IF. As for converting from the digital samples to a continuous (analog) waveform, this conversion can take place anywhere in the block diagram shown depending on technology and design choices (cost, size, performance and power dissipation). For example, there are implementations where the baseband $I$ and $Q$ samples (for the discrete baseband waveform as $I[n]+jQ[n]$) are each converted first to analog using two D/A converters and low pass filters and then passed into the block diagram above as analog waveforms. The upconversion can then be done with RFIC's such as these. Alternatively, the processing shown in the block diagram above can all be done digitally using a Numerically Controlled Oscillator and multipliers, and then after summing the products the real signal can be converted to analog with a single D/A converter and low pass or band pass filter at either the IF carrier or RF carrier.
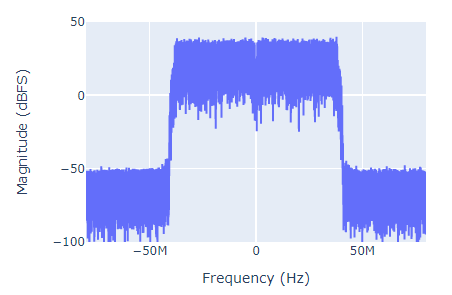
Below is a graphic representing the spectrum of an example OFDM waveform at baseband. In the frequency domain as shown, this is the FFT from the mapping of sub-carrier symbols to each bin, and results in a composite waveform spread across the occupied bandwidth shown. When translated to an IF or RF carrier as described above, we simply change the "0" representing DC in the graphic below to the new carrier frequency, and all other features would be the same; the processing above is simply a frequency translation.