Pole locations of Butterworth filter

Question

I am reading Proakis book "DSP using Matlab", 3rd edition.

I am reading chapter 8, section 8.3, p. 402, and I am confused regarding the equation of poles (roots of denominator of system function) eq 8.47.

How this equation has been derived from eq 8.46 especially highlighted line how the right most side of eq 8.47 $Ω_ce^{j(\pi/2N)(2k+N+1)}$ has been derived from left side $(-1)^{1/2N} jΩ_c$.

Answer 1

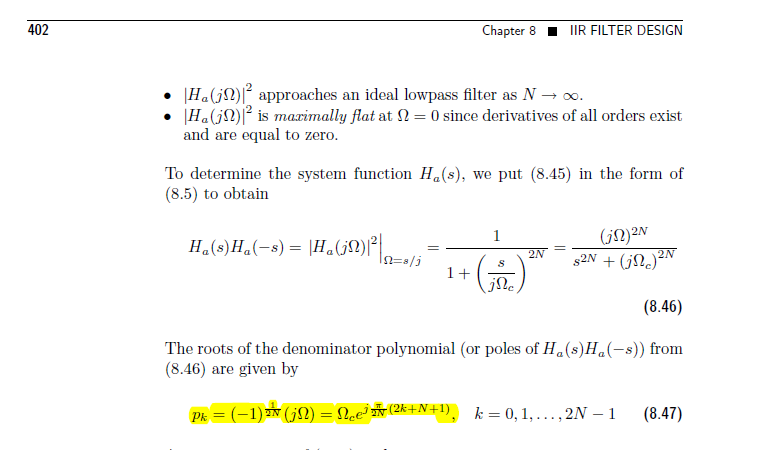
Assuming that you understand the left-hand side of Eq. $(8.47)$, for understanding the right-hand side you need to know that $-1=e^{j\pi}$, and that $e^{j2k\pi}=1$. So in order to obtain all $2N$ roots of $(-1)^{\frac{1}{2N}}$ you rewrite $-1$ as

$$-1=e^{j\pi}e^{j2\pi k}\tag{1}$$

from which you get

$$(-1)^{\frac{1}{2N}}=e^{j\frac{\pi}{2N}}e^{j\frac{2\pi k}{2N}}=e^{j\frac{\pi}{2N}(1+2k)},\qquad k=0,1,\ldots,2N-1\tag{2}$$

And since $j=e^{j\pi/2}$, the final result is

$$\Omega_cj(-1)^{\frac{1}{2N}}=\Omega_ce^{j\pi/2}e^{j\frac{\pi}{2N}(1+2k)}=\Omega_ce^{j\frac{\pi}{2N}(1+2k+N)},\qquad k=0,1,\ldots,2N-1\tag{3}$$

If this is all new to you, you should really read up on complex numbers. They are fundamental in understanding many concepts in signal processing.

---

PS: There is a typo in the equations in the book. The numerator on the right-hand of Eq. $(8.46)$ should have $\Omega_c$ instead of $\Omega$. Also, the left-hand side of Eq. $(8.47)$ should have $\Omega_c$ instead of $\Omega$.