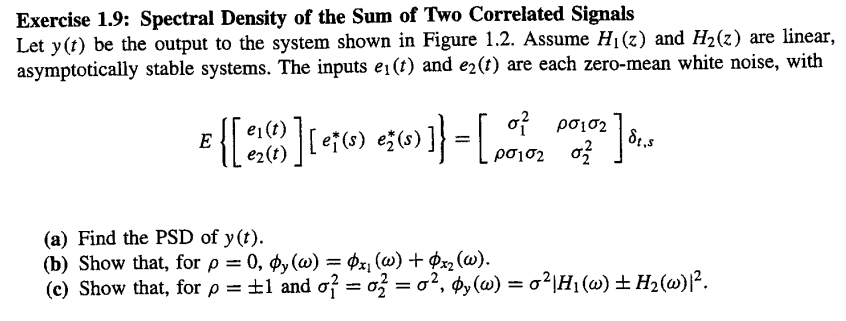PSD of the sum of two zero-mean white noise signals

Question

I am trying to solve the following exercise, where $y(t)$ is the sum of two signals $x_1(t)$ and $x_2(t)$ with each of them being the product of the convolution of $e_i(t)$ with $h_i(t)$.

So far I have found that the PSD of $y(t)$ is equal to $|H_1(\omega)|^2\sigma_1^2 + \rho\sigma_1\sigma_2[H_1(\omega)^*H_2(\omega) + H_1(\omega)H_2^*(\omega)] + |H_2(\omega)|^2\sigma_2^2$. I am stuck on (c), is there any way that I can do without the conjugates so I can get $2\rho\sigma_1\sigma_2|H_1(\omega)H_2(\omega)|$?

Answer 1

Note that for $A$ and $B$ complex, $|A|^2 = AA^*$ and $(A+B)^* = A^*+B^*$

You need to show that $$|H_1 + H_2|^2 = |H_1|^2 + |H_2|^2 + H_1^*H_2 + H_1H_2^*$$

Well:

\begin{align} |H_1 + H_2|^2 &= (H_1+H_2)(H_1+H_2)^*\\ &= (H_1+H_2)(H_1^*+H_2^*)\\ &= H_1H_1^* + H_2H_2^* + H_1^*H_2 + H_1H_2^*\\ &= |H_1|^2 + |H_2|^2 + H_1^*H_2 + H_1H_2^*\\ \end{align}