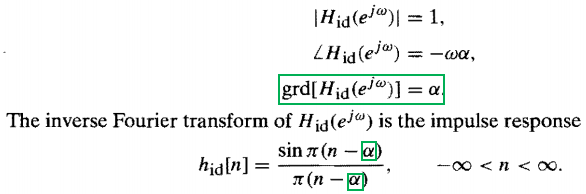In Oppenheim's Discrete Time Signal Processing there's on p. 323 no limited band in both time and frequency - wouldn't that violate the Heisenberg Principle?
$\begingroup$
$\endgroup$
2
-
$\begingroup$ what is the $grd$ operator ? $\endgroup$– AlexTPCommented Apr 29, 2017 at 9:21
-
$\begingroup$ @AlexTP: "group delay" $\endgroup$– Matt L.Commented Apr 29, 2017 at 10:32
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
2
Not at all. The Uncertainty Principle says that a function cannot be both limited in time and limited in frequency. More specifically, the product of the signal's widths in time and in frequency (i.e., its time extension $\Delta_t$ and its bandwidth $\Delta_f$) is bounded from below:
$$\Delta_t\cdot\Delta_f\ge C\tag{1}$$
where the constant $C$ depends on the definition of bandwidth and time extension.
Note that $(1)$ is a lower bound, not an upper bound, so both widths can be infinite without contradicting $(1)$.
If a function is sharply localized in time then, by the Uncertainty Principle, it cannot be sharply localized in frequency, and vice versa. However, if - as in your example - a function is NOT localized in one of the two domains then this does not mean that it must be localized in the other domain. In may very well be non-localized in both domains, as is the case in the given example for non-integer $\alpha$.
Also take a look at this question and its answers for more details on the the Uncertainty Principle.
-
2$\begingroup$ @Starhowl: The author clearly understands the principle. And my answer is very much related to your question. Your question comes from a misunderstanding of the uncertainty principle. In my answer I tried to point out that a signal with infinite time extension and infinite bandwidth clearly does NOT violate the uncertainty principle. $\endgroup$– Matt L.Commented Apr 29, 2017 at 10:31
-
4$\begingroup$ @Starhowl, your question wrongly implies that, if a signal's frequency spectrum has infinite support, than its time domain support must be finite according to uncertainty principle. As Matt L described, the principle does not say that. For signal processing applications it's interpreted in the way that a signal cannot be both time and frequency limited... So just like a Gaussian signal $x(t)=e^{-a|t|}$, it can have infinite support in both time and frequency domains. $\endgroup$– Fat32Commented Apr 29, 2017 at 11:15