Cutoff frequency condition of input signal so that it can be reconstructed , given frequency response (Answer explanation?)

Question

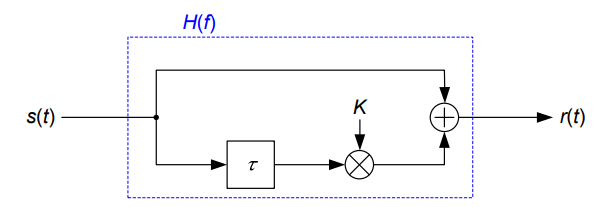
For the following system:

We have: $$r(t) = s(t) + Ks(t-\tau)$$

where $|K|< 1$, with the following impulse response $h(t)$ and frequency-response $H(f)$: $$h(t) = \delta_0(t) + K\delta_0(t-\tau)$$ $$H(f) = 1 + Ke^{-j2\pi f\tau}$$

The following question is asked:

For a band-limited input signal $s(t)$ with cutoff frequency $f_g$ (for the spectrum $S(f)$ applies $S(f)=0$ for $|f| > f_g$ ), which condition must $f_g$ satisfy, so that $s(t)$ can be completely reconstructed from $r(t)$?

When I attempt to answer this question, Shannon's sampling theorem comes to mind. The system-frequency should be greater than $2\cdot f_g$. But first, from the $H(f)$ of this system, how can I derive the systems frequency?

The answer key provides the following answer, which I don't understand:

Theoretically, there are for $|K| < 1$ no bandwidth restrictions. ($|K|= 1$ would lead to complete cancellations at discrete frequencies). In practice, however, even for $K$ values close to one, the recovery is associated with a strong increase in noise if the bandwidth $f_g$ becomes so large that the pronounced attenuation maxima of the channel are in the band occupied by $S(f)$.

Could someone provide a more detailed explanation? Why are there in principle theoretically no restrictions?

Answer 1

Hint: Assume $K = 1$. Is there a signal $s(t) \neq 0$ that produces an output equal to zero? You would need a signal such that, when delayed, is equal to its negative: $s(t) + s(t-\tau) = 0$.

Bonus question: would such an $s(t)$ be periodic necessarily?

Hint 2: Why did we special-cased $K = 1$ above? What happens if $K \neq 1$?