Confusion understanding phase shift/delay?

Question

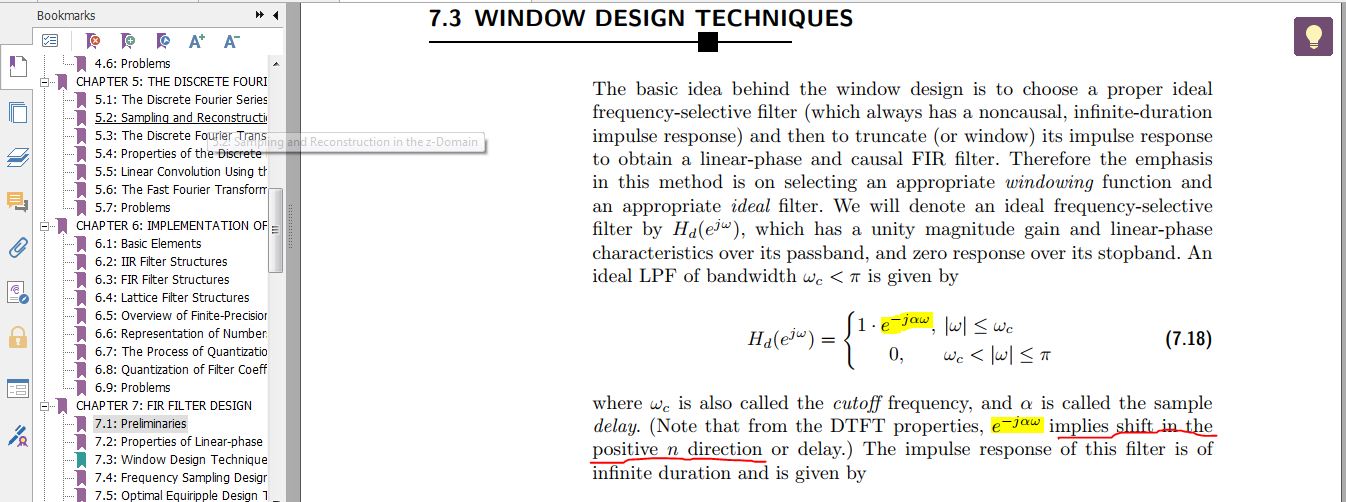
I am reading proakis, as shown highlighted in attached snap shot,there is '-'sign along with j,but still it is written (underlined red) implies shift in positive n direction,why and how positive?when we have negative sign with j?

Answer 1

Delay is the negative derivative of phase with respect to frequency. Phase that increases linearly negative as frequency increases will result in a positive (later) delay in time. This is given by the Fourier Transform property of time delay:

$$\mathscr{F} \{f(t-\tau)\} = e^{-j\tau \omega}F(\omega)$$

And is easy to prove from the Fourier Transform:

$$\mathscr{F} \{f(t-\tau)\} = \int_{-\infty}^\infty f(t-\tau)e^{-j\omega t}dt$$

$$ = \int_{-\infty}^\infty f(t-\tau)e^{-j\tau \omega}e^{j\tau \omega}e^{-j\omega t}dt$$

$$ = e^{-j\tau \omega}\int_{-\infty}^\infty f(t-\tau)e^{-j\omega (t-\tau)}dt$$

If we substitute $u=t-\tau$, then $du = dt$ and we get:

$$ = e^{-j\tau \omega}\int_{-\infty}^\infty f(u)e^{-j\omega u}du =e^{-j\tau \omega}F(\omega) $$

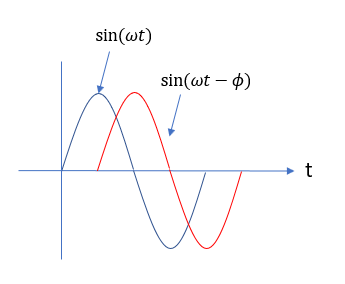
Also without going into the details of the Fourier Transform, consider how we subtract phase in the time domain to shift a sine wave in time to the right: