Confusion regarding sinusoidal synthesis formula,

Question

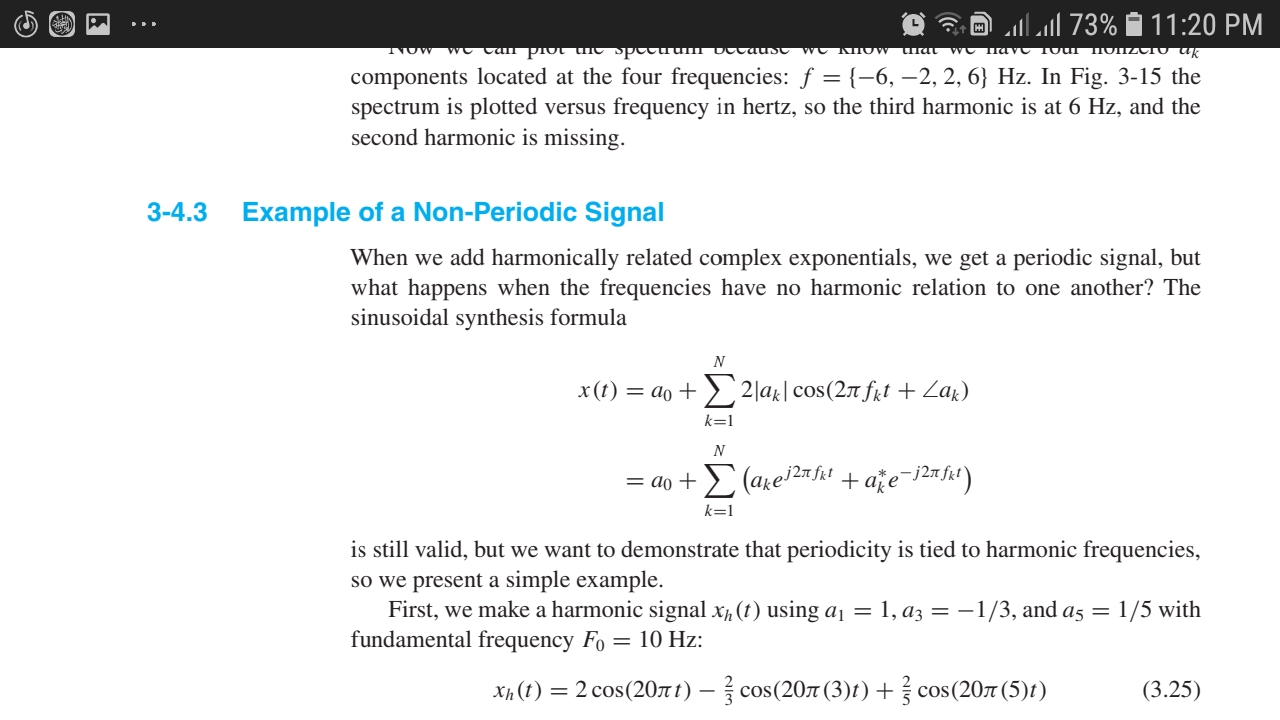
I am reading signal processing first by McClellan

As shown in attached snapshot, we have formula of sinusoidal synthesis

I am confused ,why in expression of x(t), there is a '2' in first line immediately after summation sign but that'2' disappear in 2nd line?

Answer 1

The second term can be expanded to $$\begin{align} 2|a_k|\left(\frac{ e^{i(2\pi f_k t+\angle a_k)} + e^{-i(2\pi f_k t+\angle a_k)}}{2}\right) &= |a_k|e^{i\angle a_k}e^{i(2\pi f_k t)}+|a_k|e^{-i\angle a_k}e^{-i(2\pi f_k t)} \\ &= a_k e^{i(2\pi f_k t)}+a_k^*e^{-i(2\pi f_k t)} \\ \end{align}$$

Since $a_k=|a_k|e^{i\angle a_k}$ and $a_k^*=|a_k|e^{-i\angle a_k}$ and $\cos\theta=\frac{ e^{i\theta} + e^{-i\theta}}{2}$

Answer 2

Simple:

Euler's formula:

$$ e^{i\theta} = \cos(\theta) + i \sin(\theta) $$

and

$$ e^{-i\theta} = \cos(\theta) - i \sin(\theta) $$

Add them together:

$$ e^{i\theta} + e^{-i\theta} = 2 \cos(\theta) $$

You should see that in your equation.

From there it is usually stated:

$$ \cos(\theta) = \frac{ e^{i\theta} + e^{-i\theta}}{ 2 } $$

See my article

• The Exponential Nature of the Complex Unit Circle

for a more expansive explanation.