I was asked this question in an interview couple of weeks ago. I think I answered it correctly, but I think I might missing some points. Here is the problem, following by my answer.
Part 1)
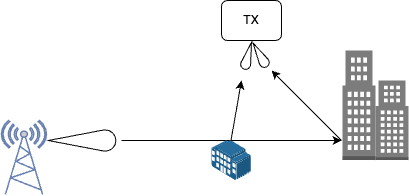
In Figure 1, for one of the TX beam, we have two of the strongest RX beams, $V_1$, $V_2$, obtain frequency-flat complex channel estimation of $h_1$ and $h_2$. Other RX beams receive no signal.
- What is the optimal RX beam for best performance?
Figure 1
Part 2)
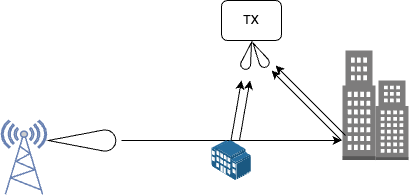
Figure 2
Now let's assume for one of the TX beam, two of orthogonal strongest RX beams, $V_1$, $V_2$, obtain two channel responses of $\mathbf{h}_1$ and $\mathbf{h}_2$, respectively. Other RX beams receive no signal. Channel response has $K$ taps and can be written as $\mathbf{h}_1 = (h_1[0],h_1[1]\dots,h_1[K-1])$ and $\mathbf{h}_2 = (h_2[0],h_2[1]\dots,h_2[K-1])$
- What is the optimal RX beam for best performance?
Answer: For both parts, we do Maximum Ratio Combining to get he optimal RX beamform for the best performance (here maximizing the received SNR), assuming $h_1$ and $h_2$ are obtained. One can write the SNR as $SNR = \frac{|w^H * x|^2}{(w^H * N)}$ where after maximizing this term, we can see MRC is the solution.
However, I think there could be more to this problems. Can this be done using any other beamforming methods to get the best performance (based on maximizing SNR)?