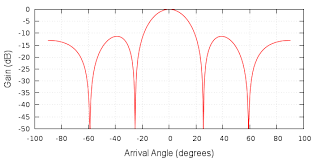
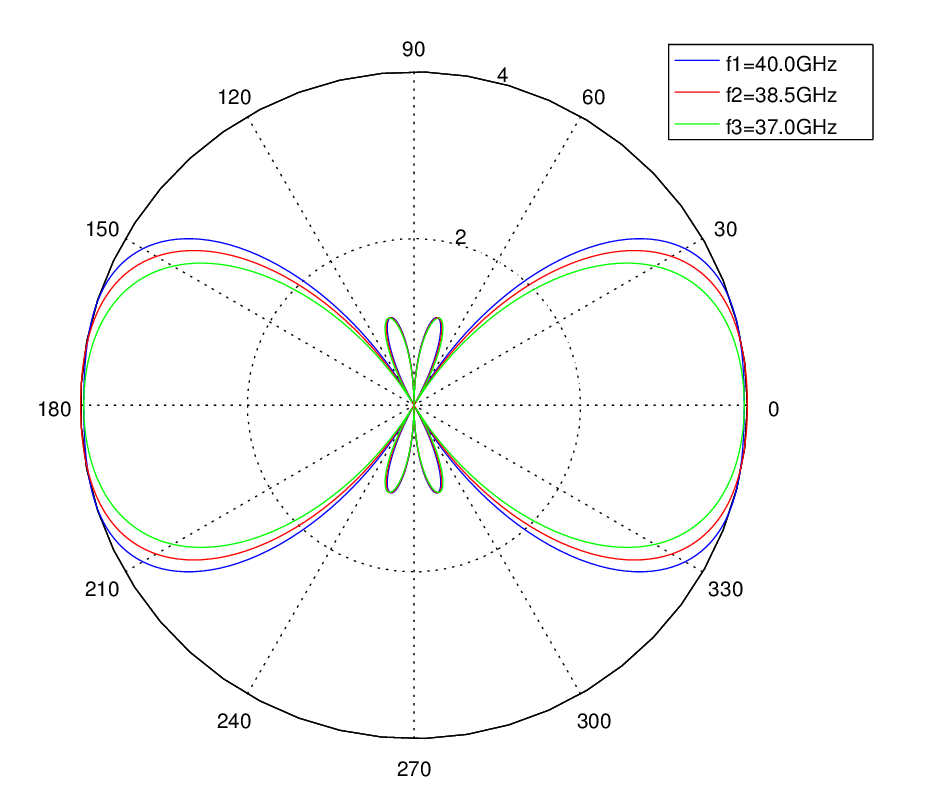
the plots are likely from a line or what is often called a ULA and there is a mirror image response. It is sometimes called a left/right ambiguity.
Your question’s answer depends on the purpose of your simulation. The plots are probably equivalent, the response is over 360 degrees in both plots but people often drop the redundant half circle.
if you have a problem where objects are distributed over 360 degrees, simulate that, otherwise 180 is fine.
I usually plot in $\cos(\theta)$ coordinates which range from -1 to 1 for most of my work. For a ULA, the pattern is the same regardless of the steering direction using this convention. If the array weights are symmetric, 0 to 1 is sufficient.
It all depends on what your application is and what you are investigating and the conventions of your community which isn’t specified in your question.
The only caveat is that in some applications you might have some structure blocking the back half of the array. In SONAR it is called a baffle. In RF there are other caveats like a ground plane on one side.
Actual arrays have more complicated responses because what you showed are probably Array Factor responses where each element is assumed ideally “isotropic”. Real array elements have some directionality. The Array Factor also ignores element to element coupling.
In summary, it could be warranted but in many applications it might not matter.