My question is on OFDM signals for LTE. I been reading a tutorial on LTE where ofcourse the modulation is OFDM and it is explained that an OFDM signal is a rect function in time domain. The duration is equal to $\frac{1}{\Delta f}$ assuming ${\Delta f}$ is the subcarrier spacing. Then it is explained that in frequency domain the subcarriers will have sinc func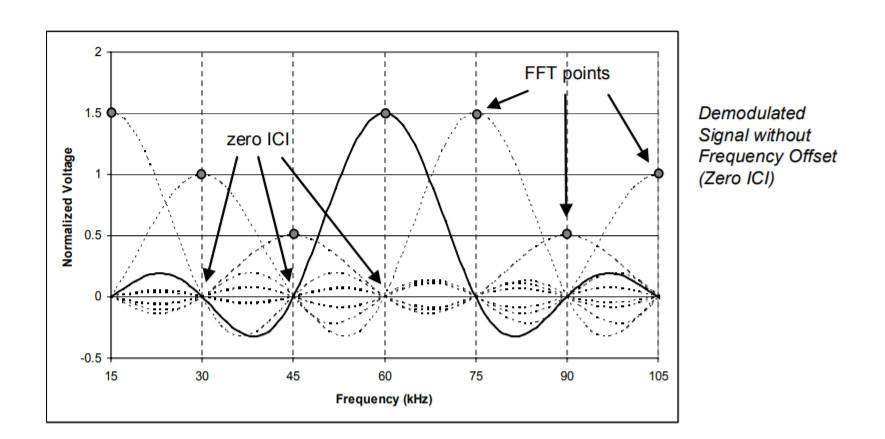 (see figure above) and that the crossings are at integer multiples of the subcarrier frequencies $k \Delta f$
(see figure above) and that the crossings are at integer multiples of the subcarrier frequencies $k \Delta f$
I was then looking for the Power spectral density of OFDM signal. And somewhere it is mentioned that it should look like this 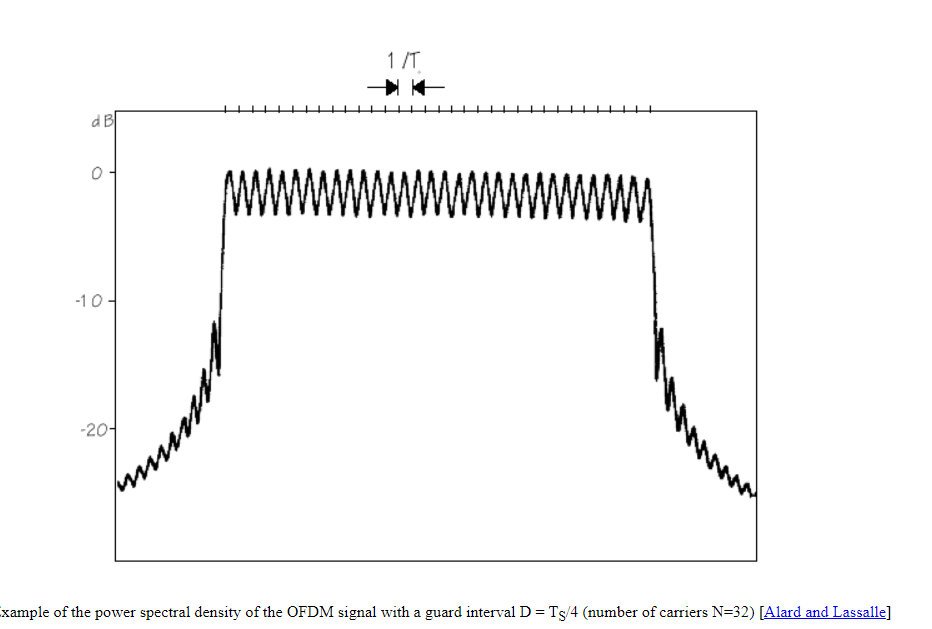
I have no idea why the PSD of sinc function will end up being looking like what is presented in the second figure. Isnt the PSD just the absolute value of the signal in frequency domain squared?
How do you go from sinc to this PSD. Hope someone can help.
Thanks,
