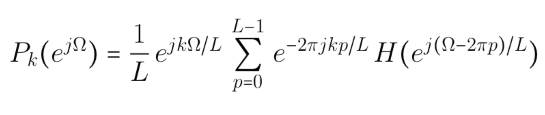Let's start with your equation.
$$ P_k\left( e^{j\Omega} \right) = \frac{1}{L} e^{jk\Omega/L} \sum_{p=0}^{L-1} e^{-2 \pi j k p / L} H\left( e^{j(\Omega-2\pi p)/L} \right) $$
Do a little rearranging.
$$ P_k\left( e^{j\Omega} \right) = e^{j\Omega\frac{k}{L}} \frac{1}{L} \left[ \sum_{p=0}^{L-1} H\left( e^{j\Omega\frac{1}{L} } e^{-j2\pi \frac{p}{L}} \right) \left( e^{j2\pi/L} \right)^{-kp} \right] $$
It can be made simpler to understand by a few variable substitutions.
$$ y = e^{j\Omega} $$
$y$ is a complex value on the complex unit circle at $\Omega$ radians around the circumference.
$$ h[p] = H\left( y^{\frac{1}{L}} e^{-j2\pi \frac{p}{L}} \right) $$
$h[]$ is a sequence of values sampled from the $H()$ function. $y^{\frac{1}{L}}$ is a complex value on the unit circle at $1/L$th the angle of $y$. The $e^{-j2\pi \frac{p}{L}}$ is a clockwise walk around the unit circle in $L$ even steps. Conceptually:
$$ e^{-j2\pi \frac{p}{L}} = \left( e^{j2\pi } \right)^{-\frac{p}{L}} = 1^{-\frac{p}{L}} $$
Therefore $h[]$ has a period of $L$. If $p$ were to go below zero or above $L-1$, it would be a repeat pattern.
With these substitutions, your equation becomes this:
$$ P_k\left( y \right) = y^{\frac{k}{L}} \frac{1}{L} \left[ \sum_{p=0}^{L-1} h[p] \left( e^{j2\pi/L} \right)^{-pk} \right] $$
The part in brackets is the definition of the DFT.
$$ X[k] = \sum_{n=0}^{N-1} x[n] \left(e^{i2\pi/N}\right)^{-nk} $$
It's arranged the same way as in my answer here Fractional powers of complex numbers (DSPrelated computation)
The $\frac{1}{L}$ is a normalizing factor making the magnitudes of the DFT calculation independent of $L$.
$ y^{\frac{k}{L}} $ is a complex value $ k/L $ of the way to $y$ along the unit circle which means the results of the DFT bin are rotated that far.
So, a long answer to your question. The purpose of the second exponential $ e^{-2\pi j k p/L} $ is to execute the DFT transform. It is part of the definition and the reason it works the way it does. Lots of stuff available on that if you are unfamiliar.