1
$\begingroup$

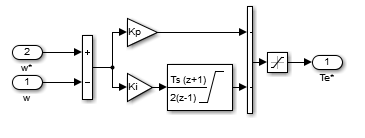
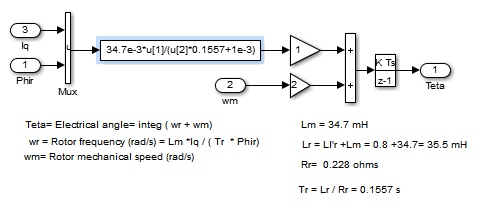
I am an electrical engineering student but new to digital signal processing. However while searching references for my senior project I came across following two different discrete integrator blocks in a MATLAB - Simulink simulation and I can't identify what these blocks do. I am familiar with continuous time integrators but this is quite new thing for me.

enter image description here

The above block - Ts(z+1)/2(z-1) is discrete time integrator with upper and lower saturation limits defined and that's all I got to know about upon clicking on it, however I want to know what does exactly that (z+1) and (z-1) means and how is it different from one shown below (KTs/(z-1)):

enter image description here

To the best of my knowledge above integrator is one which is direct discrete counterpart of continuous time integrator (correct me if I'm wrong), essentially Ts means it multiplies sampling time as essential in integration and 1/z-1 is inverse z-transform of a[k] = -u[-k] , right? But even if that's right, I don't understand what that term is doing here.

If my that understanding is right, what does the integrator in first block wants to tell me?

Also, the integrator in first picture is a PI torque controller, it produces reference torque command based on speed error and so that integrator is "I" block of "PI", I think but why is it different from the one in last pic. The one in last pic just... 'integrates'...much like it's continuous time counter part.

Thanks in advance and bear with my, I'm very new to this stuff.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

There are different methods to approximate integration in discrete time. The most straightforward ones are the forward and backward Euler methods, and the trapezoidal method. A discrete-time system with transfer function

$$H(z)=\frac{T}{z-1}\tag{1}$$

implements the forward Euler method.

Similarly, the backward Euler method is implemented by

$$H(z)=\frac{Tz}{z-1}\tag{1}$$

Finally, the trapezoidal method is implemented by

$$H(z)=\frac{T}{2}\frac{z+1}{z-1}\tag{1}$$

These discrete-time integrators are also described on this mathworks page.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Tysm for your answer. Are there any thumb rules that when to use which? $\endgroup$
    – Deep
    Commented Sep 17, 2018 at 10:19
  • $\begingroup$ @Deep: I don't think so, you just have to see what works best for your specific application. $\endgroup$
    – Matt L.
    Commented Sep 17, 2018 at 10:29

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.