0
$\begingroup$

s

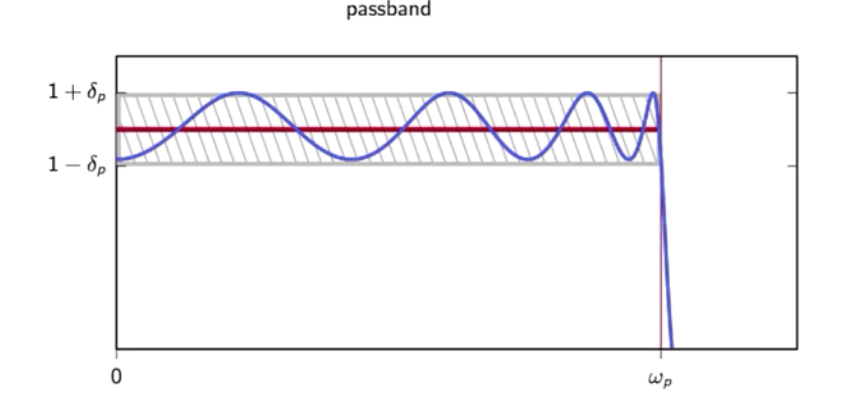
The teacher says As a consquence, you can think of the frequency response of a filter as a shark. It must always move and it can never be rest. An important case is equiripple.

jj

I am very confused about the abrupt conception..

  1. Why constant frequency response can never be rest?
    2.how does constant frequency response relate to equiripple?

Thanks you!!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

What your teacher tried to show is that a transfer function which is the ratio of two polynomials can never be constant (excluding the trivial case where both polynomials are of degree zero). This means that if your desired frequency response is a piecewise constant function (as is the case for an ideal frequency selective filter), you can only approximate that piecewise constant function by a realizable transfer function, and one popular approximation is an equi-ripple approximation, where the maximum deviation from the desired constant value is minimized.

Improve this answer
$\endgroup$
3
  • $\begingroup$ In a short time interval, the devation of the equi-ripple approximation is large. Do you mean that over the whole interval? $\endgroup$
    – user22971
    Commented Jul 27, 2016 at 9:15
  • $\begingroup$ @user22971: We're talking about the frequency domain, so there's no time interval. An equi-ripple approximation means that the maximum deviation (i.e., at the frequencies where you have local maxima or minima of your approximating function) is minimized. All other types of approximation will have a larger maximum error. $\endgroup$
    – Matt L.
    Commented Jul 27, 2016 at 9:40
  • $\begingroup$ Eventhough I agree on the answer of showing "why a an nth degree polynomial with a constant value" is impossible to realize in finite degree other than n=0, from the point of filter design the monotonic (albeit nonconstant) approach, without ripples, should also be included to complement the idea, to emphasize the fact that the equiripple error is not a necessity but a choice for obtaining certain characteristics such as minimum of maximum error (i.e. minimax) over the entire band of interest. $\endgroup$
    – Fat32
    Commented Jul 27, 2016 at 14:29

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.