2
$\begingroup$

While studying an OFDM system, I have encountered statements like coherence bandwidth is the reciprocal of delay spread and coherence time is the reciprocal of the Doppler spectrum.

Can somebody help me to understand this mathematically?

How is coherence bandwidth related to delay spread, and how is coherence time related to Doppler spread?

Improve this question
$\endgroup$
6
  • $\begingroup$ I can visualize, DFT of a Kronecker Delta Function will be all ones, So if my delay spread is less [time domain] then coherence bandwidth will be more. $\endgroup$
    – rajez79
    Commented Oct 29, 2013 at 2:59
  • $\begingroup$ Coherence Bandwidth is a statistical range of frequencies over which my channel is considered flat. If two sinusoids seperated by a distance of Bc in frequency will be affected differently by the channel, but my delay spread is the time diffrence between the LOS component and the last received multipath component. With this understanding how can I relate Coherence Bandwith and Delay Spread. $\endgroup$
    – rajez79
    Commented Oct 29, 2013 at 3:03
  • $\begingroup$ If my delay spread is more then my coherence bandwidth will be less, based on this only my pilot positioning will be done, so can I have less number of pilots in my OFDM symbol in this case ? Please comment on this. $\endgroup$
    – rajez79
    Commented Oct 29, 2013 at 3:06
  • $\begingroup$ Again the same quiestions in the case of Coherence Time and Doppler Spread. $\endgroup$
    – rajez79
    Commented Oct 29, 2013 at 3:07
  • $\begingroup$ Coherence Time is flatness in time, means the phase will be predicatable or channel impulse repsonse is not varying. Doppler spread is sprectral broadening of the channel. Am not getting how these two terms are related. $\endgroup$
    – rajez79
    Commented Oct 29, 2013 at 3:16

3 Answers 3

Reset to default
3
$\begingroup$

This diagram (from Molisch) shows the various Fourier relationships between measurements in wireless systems.

enter image description here

Improve this answer
$\endgroup$
0
3
$\begingroup$

I'm coming in pretty late, but...

Are you looking for a somewhat intuitive explanation since you already have the basic mathematical definitions?

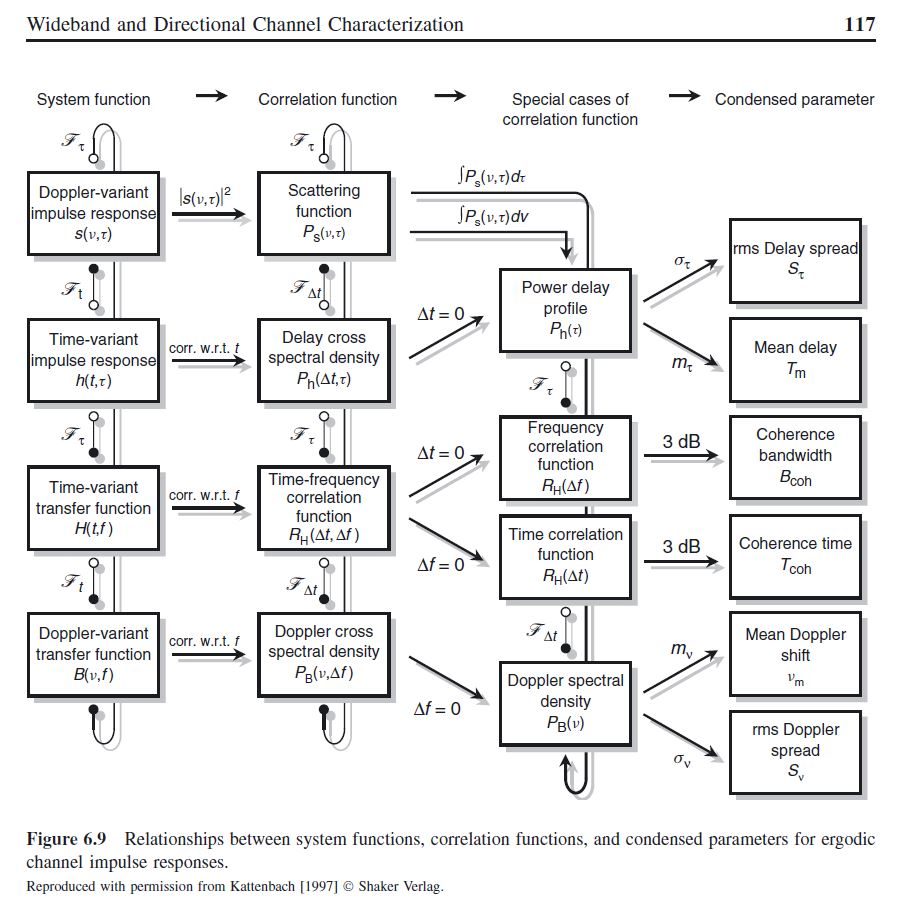
Coherence time is the time over which the channel impulse response does not change appreciably or decorrelate (with "appreciably" having different interpretations depending on what you're doing). The general idea is that if you are equalizing the channel in the receiver, the equalizer coefficients will not need to change significantly over the coherence time. If training intervals are required, the training intervals need to happen faster than the coherence time would indicate.

Likewise coherence bandwidth, for an OFDM system in particular, suggests that the frequency-domain equalizer coefficients over certain bandwidth spans will be similar. This is consistent with the definition you cited that the channel is essentially flat over the coherence bandwidth.

Both of these are statistical measures, however, as either can change quickly in a real channel and things like the spacing of nulls in the frequency domain is generally not constant.

The frequency response of the channel is the FT of the time-domain impulse response. So "coherence bandwidth" is related to the FT of the delay spread, and that delay spread is expected to stay correlated with itself over the length of the coherence time.

Hope that helps a bit.

Improve this answer
$\endgroup$
0
$\begingroup$

Similarly, the doppler spread is the inverse of the coherence bandwidth, which means having a high coherence bandwidth will lead to small doppler spread!

Improve this answer
$\endgroup$
1
  • $\begingroup$ Hi. Would you mind elaborating on that? $\endgroup$
    – jojeck ♦
    Commented Nov 9, 2017 at 12:56

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.