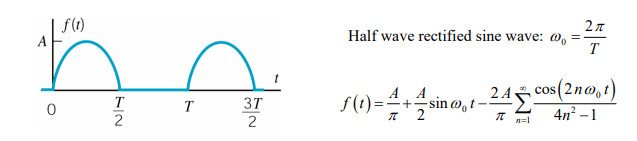Autocorrelation function of a filtered periodic signal

Question

I'm having trouble with this exercise. The signal $x(t)= \cos(2\pi f_0 t)$ is sent in input to a non-linear system with input-output relationship equal to: $g(x)= 0$ if $x<0$ and $g(x)=3x$ if $ x >= 0$.

1. Find $y(t)$ and its Fourier transform. The output of this non-linear system is $y(t)$ and can be written as $$ y(t)= \sum_{k=-\infty}^{+\infty} {\cos(2\pi f_0 t) \cdot rect(\frac{t-kT_0}{\frac{T_0}{2}})} $$ . Using the Fourier series, we have $y(t)= \sum_{k=-\infty}^{+\infty} Y_k \cdot e^{j2 \pi f_0 kt}$ , where $Y_k= \frac{1}{T_0} \cdot \int_{-\frac{T_0}{2}}^{\frac{T_0}{2}} {y(t) \cdot e^{-j2 \pi f_0 kt} dt}= \frac{3}{4} \cdot [sinc(kf_0 \cdot \frac{T_0}{2} - f_0) + sinc(kf_0 \cdot \frac{T_0}{2} + f_0)] = \frac{3}{4} \cdot [sinc(\frac{k}{2} - f_0) + sinc(\frac{k}{2} + f_0)] $ We transform then $y(t)$ and we obtain $Y(f)$. Alternatively, we can see $y(t)$ as $y(t)= 3 \cos(2 \pi f_0 t)rect(\frac{t}{\frac{T_0}{2}}) * \sum_{n=-\infty}^{+\infty}{\delta(t-nT_0)}$ and transform this to get $Y(f)$. So we have that $Y(f)= \sum_{k=-\infty}^{+\infty} {Y_k \cdot \delta(f-kf_0)}$. Is there any mistake so far (are $y(t)$ and $Y(f)$ correct)?

2. $y(t)$ is sent in input to a High pass filter with cutoff frequency equal to $2.5 f_0$ . Find the output $z(t)$ and calculate its autocorrelation function $R_x (\tau)$. Said filter has the frequency response equal to $$H_{HP} (f) = 1-rect(\frac{f}{2B}) = 1-rect(\frac{f}{5f_0})$$ , right? How do I calculate te autocorrelation function of $z(t)$?

In the frequency domain we have $$Z(f)= \frac{3}{4} \cdot \sum_{k=-\infty}^{-3} {[sinc(\frac{k}{2} - f_0) + sinc(\frac{k}{2} + f_0)}] \cdot \delta(f-kf_0) $$ $$ + \frac{3}{4} \cdot \sum_{k=3}^{+\infty} {[sinc(\frac{k}{2} - f_0) + sinc(\frac{k}{2} + f_0)] \cdot \delta(f-kf_0)} $$. Am I correct? Should antitransform $Z(f)$ and then calculate the autocorrelation function?

The definition of Autocorrelation Function is $R_z(\tau)= \int_{-\infty}^{+\infty} {z(t) \cdot z(t- \tau)} dt$ . Theoretically we can look at it as a convolution product between $x(t)$ and $x(-t)$ ; considering that $z(t)$ is a power signal, $R_z(\tau)= lim_{T \longrightarrow \infty} \frac{1}{T} \cdot \int_{-\frac{T}{2}}^{+\frac{T}{2}} {z(t) \cdot z(t- \tau)} dt $ and in the frequency domain it becomes the Power Spectral Density of the signal ($ S_z(f)= lim_{T \longrightarrow \infty} \frac{|Z(f)|^2}{T} $). Is everything stated until now correct?

Answer 1

(Note to others: Please don’t edit this yet as OP and I are still working through certain details to make this clearer… once this not is gone, feel free to make updates )

This was a (advanced) homework problem with a full solution developed below, but the OP has gone through this with me thoroughly to develop the final result shown below which was a useful and learning exercise for the both of us.

Verification of Part 1 (with iterative help from the OP)

My approach would be to compute the Fourier Transform extending over one period of the waveform $y(t)$, extending from $-T_o/2$ to $+T_o/2$ with $f_o= 1/T_o$, knowing then that the Fourier Transform of the periodic repetition of that waveform will be the same result but only existing at $k f_o$ for any integer $k$. (So we find the envelope using the FT of $y(t)$ over the one period and then sample that function at the discrete frequency locations given by $kf_o$, which is then the Fourier Series.)

With that approach the Fourier Series would become:

$$Y_k = f_0\int_{t=-1/(2f_o)}^{1/(2f_o)} 3\cos(2\pi f_o t)\Pi(2f_o t)e^{-j2\pi kf_ot}dt \tag{1} \label{1}$$

for $k \in \mathbb{Z}$ (the set of all integers $-\infty$ to $+\infty$).

Due to the rect function, we can simplify by restricting the integration limits according to the rect:

$$Y_k = f_0\int_{t=-1/(4f_o)}^{1/(4f_o)} 3\cos(2\pi f_o t)e^{j2\pi kf_ot }dt\tag{2} \label{2}$$

Further, since the time domain waveform is an even function, the result will be real and the FT calculation simplifies to the following equation since $$e^{j2\pi kf_ot} = \cos(2\pi kf_ot)+j\sin(2\pi kf_ot)$$ and the $j\sin(2\pi kf_ot)$ will integrate to 0 in this case, resulting in:

$$Y_k = 3f_o\int_{t=-1/(4f_o)}^{1/(4f_o)} \cos(2\pi f_o t)\cos(2\pi kf_ot )dt\tag{3} \label{3}$$

So we have:

$$Y_k = \begin{cases}3f_o\int_{t=-1/(4f_o)}^{1/(4f_o)} \cos(2\pi f_o t)dt,& k=0\\3f_o\int_{t=-1/(4f_o)}^{1/(4f_o)} \cos(2\pi f_o t)\cos(2\pi kf_ot )dt,&k \neq 0\end{cases}\tag{4} \label{4}$$

To solve the definite integral, it helps to use the trigonometric identity for a product of cosines, which results in equation $\ref{4}$ being equal:

$$Y_k = \frac{3f_o}{2}\int_{t=-1/(4f_o)}^{1/(4f_o)}\cos(2\pi f_o (1+k)t)dt + \frac{3f_o}{2}\int_{t=-1/(4f_o)}^{1/(4f_o)}\cos(2\pi f_o(1-k) t) dt $$

The rest of the tedious intermediate steps not shown for solving the integral, but the final result of this is: $$Y_k = \begin{cases}\frac{3}{\pi}, & k=0\\\frac{3\cos(\pi k/2)}{\pi(1-k^2)}, &k>0\end{cases}\tag{5} \label{5}$$

Given integer $k$, equation \ref{5} reduces to:

$$Y_k = \begin{cases}\frac{3}{\pi},&k=0\\\frac{3}{4}, &|k|=1\\0, &k> 1 \text{ and k odd}\\(-1)^{k/2}\frac{3}{\pi(1-k^2)}, & \text{k even}\end{cases}\tag{6} \label{6}$$

As confirmation, this result is consistent with this table by Professor Jim Svoboda of Clarkson University for the Fourier Series of common waveforms, with the half wave rectified sine wave (instead of cosine here) given in the graphic copied below:

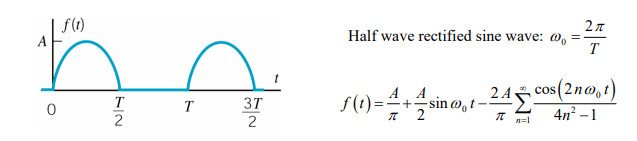
As an alternate approach, we can use the product in time is convolution in frequency and get the results for each $Y[kf_o]$ as the Fourier Transform given by the convolution of the FT of $3\cos(2\pi f_o t)$ and the FT of $\Pi(2f_o t)$, sampled at integer multiples of $f_o$. This results in:

$$Y[kf_0] = (X_1(f) * X_2(f))\delta(f-kf_o)\tag{7} \label{7}$$ with:

$$X_1(f)=\mathscr{F} \{3\cos(2\pi f_o t) \}= \frac{3}{2}\delta(f-f_o)+ \frac{3}{2}\delta(f+f_o)\tag{8} \label{8}$$

$$X_2(f)= \mathscr{F} \{\Pi(2f_o t) \}= \text{Sinc}(f/(2f_o)\tag{9} \label{9}$$

Proceeding with that approach:

$$X_1(f) * X_2(f) = \frac{3}{2}\text{Sinc}\bigg(\frac{f-f_o}{2f_o}\bigg) + \frac{3}{2}\text{Sinc}\bigg(\frac{f+f_o}{2f_o}\bigg)\tag{10} \label{10}$$

Given the result above is sampled at integer multiples of $f_o$, this can be simplified given the relationship that the magnitude of $Sinc(k/2)$ intersects with the magnitude of $2/(\pi k)$ at integer $k$ as depicted in the following graphic:

$$|\text{Sinc}(k/2)| = \bigg|\frac{2}{\pi k}\bigg|, \text{ for odd integer k} \tag{11} \label{11}$$

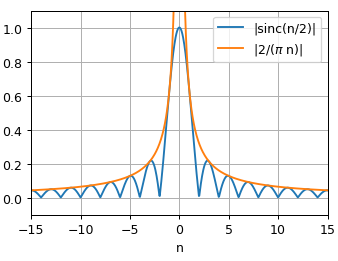
And further, the Sinc function alternates in sign for each alternate odd $k$, resulting in:

$$\text{Sinc}(k/2) = (-1)^{(k-1)/2}\frac{2}{\pi k}, \text{ for odd integer k} \tag{12} \label{12}$$

Thus equation \ref{7} becomes:

$$Y[kf_o] = \frac{3}{2}\text{Sinc}\bigg(\frac{f-f_o}{2f_o}\bigg)\delta(f-kf_o) + \frac{3}{2}\text{Sinc}\bigg(\frac{f+f_o}{2f_o}\bigg)\delta(f-kf_o)$$

Which is then simplified using the relationship given in Equation \ref{12} which should then match equation \ref{6} (to be verified).

Verification of Part 2

The high pass filter simply blocks $Y_k$ for $|k| \le 2$ and passes $|k| >2$, (for all $k\ge 0$) to create $Z_k$, so from equation \ref{6}:

$$Z_k = \begin{cases} (-1)^{k/2}\frac{3}{\pi(1-k^2)}, & |k|>3\text{ and k even}\\0 &\text{ otherwise}\\\end{cases}\tag{13} \label{13}$$

Since $z(t)$ is a periodic signal, its Autocorrelation Function is periodic too and thus has its own Fourier Series. $$R_z(\tau)= \sum_{k=-\infty}^{+\infty} {|Z_k|^2 \cdot e^{j2 \pi f_0 k \tau}} = 2 \cdot \sum_{p=2}^{+\infty} {(-1)^p \cdot \frac{9}{(\pi)^2 \cdot (1-4p^2)^2} \cdot \cos(4\pi p f_0 \tau)}$$ $$ = \frac{18}{(\pi)^2} \cdot \sum_{p=2}^{+\infty} {\frac{(-1)^p}{1-4p^2} \cdot \cos(4 \pi p f_0 \tau)} $$