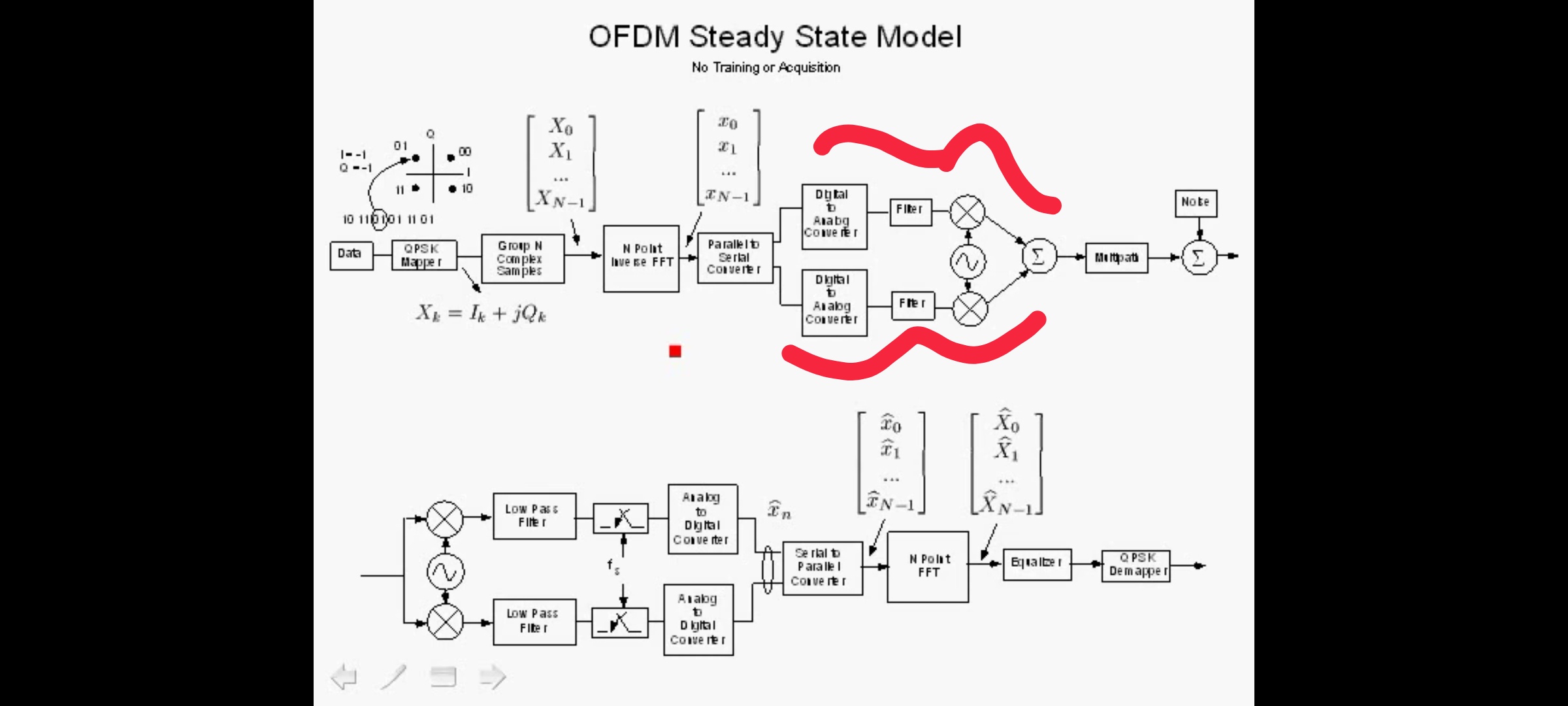
OFDM Basic Query- x[n] produced at output of IFFT is in discrete time, so we can use DAC and then RF Upconversion (multiply it with LO Frequency say f) and send it, why are we doing RF up conversion in Inphase and Quadrature phase then adding it and sending it? Can someone please explain.
Because the output of the IFFT operation is a vector of complex numbers. An RF signal is a real thing, so you can't just multiply a sine wave by a complex number and get something sensible.
However, you can map a complex number onto an RF carrier by multiplying the real part by $\cos \omega t$, and the imaginary part by $\sin \omega t$. This is I/Q modulation. The result is not complex -- it's all real. But the resulting arithmetic that you do on it, at the transmit and the receive end, is the same as if it were a complex number.
That's the beauty and convenience of I/Q modulation and demodulation.
Each IFFT complex result bin represents an amplitude and a phase for some frequency. You can use the sum of the a sine and a cosine in the appropriate ratios to produce a sinusoid of any phase (see trig identity). The IQ inphase and quadrature modulators produce the sine and cosine in the appropriate ratios to produce the transmit phase as needed.
This allows producing a phase modulated output using 2 non-modulated (thus simpler and cleaner) oscillators (or one oscilator with a quadrature output). (at the added cost of 2 modulators plus a summing node or circuit)
