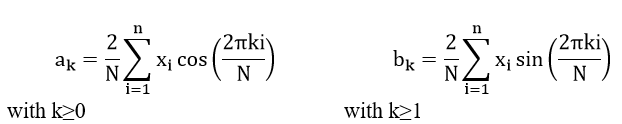Amplitude in frequency domain of the same signal measured at different bandwidth (f_max - f_min) and different FFT lines

Question

Fourier series in complex form

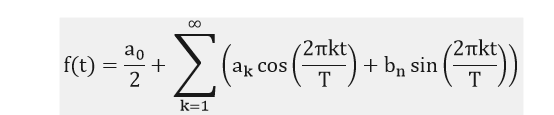
Fourier series in trigonometry form

---

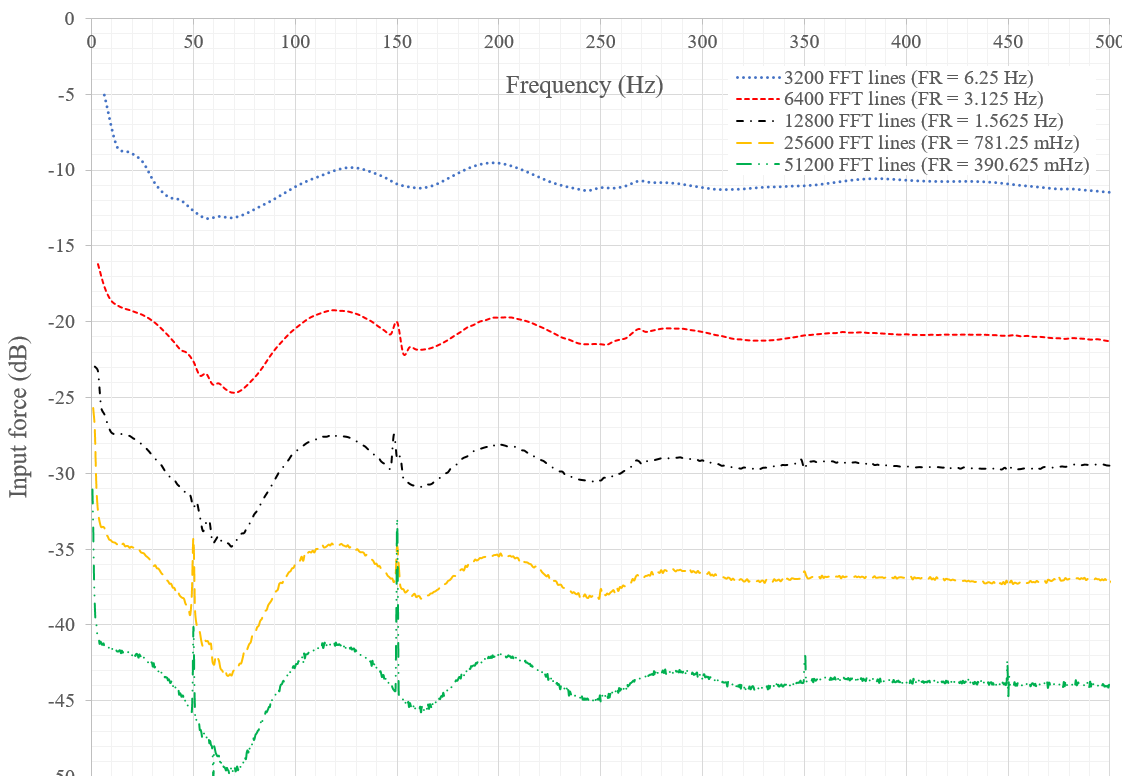
Here, I'm measuring the same signal (an impulse force - or at least as close as possible to an impulse, represented by a knocking force). The work I'm doing is parameter study (i.e.: studying the effect of different bandwidths and numbers of FFT lines).

This is on the same number of FFT lines but different bandwidth

And this is the same bandwidth and different FFT lines

The main conclusion I can draw is that changing one parameter (while keeping the other is the same) would change the amplitude of the frequency spectrum. I can explain this via math above (smaller frequency resolution leads to longer measuring time, and thus lower Fourier coefficients).

But what does it mean in a practical and realistic manner? As in for actual sampling process, how would changing the parameters (measuring time, sampling points...) affect the frequency spectrum of the same signal?

Edit: The smaller frequency resolution would eventually lead to lower amplitude in frequency spectrum is considered as analytical math by my instructor, while the actual system works with numerical math. So a more "physical" (or "what actually happens") answer is needed.

Answer 1

Are you using the correct FFT form for spectral analysis? You need to divide by N when mapping time->frequency domain with an N-point FFT for a power spectrum. If you want power spectral density you have to factor in the bin bandwidth and window effective noise bandwidths.

The amplitude of a spectral line represents the power of a single signal frequency, power spectral density reflects power per unit bandwidth, which is different.