Darkling, these things are quite clearly explained in standard signals & systems textbooks. But I assume you have little time left to read (as most undergraduate courseware are full of homeworks, preliminaries, and projects that most of which I believe become harmful as they consume a student's learning time; and just completing a homework or project without full understanding makes what sense..? anyway)
Consider the LCCDE associated with a causal LTI system (such a system is said to be at initial rest which assures that all the initial conditions are zero; i.e., no output occurs before an input is applied) :
$$ \sum_{k=0}^{N} a_k ~y[n-k] = \sum_{k=0}^{M} b_k ~ x[n-k] $$ The order is $\max\{M,N\}$.
Now the LCCDE can be considered to be a cascade of two LTI systems; the LHS represents an IIR-LTI system represented by $A(z)$ and RHS represents an FIR-LTI system represented by $B(z)$. So the system can be represented with the following block diagrams:
$$ x[n] \rightarrow \boxed{ B(z) } \rightarrow v[n] \rightarrow \boxed{ \frac{1}{A(z)} }\rightarrow y[n] \tag{1}$$
or equivalently
$$ x[n] \rightarrow \boxed{ \frac{1}{A(z)} } \rightarrow v[n] \rightarrow \boxed{ B(z) } \rightarrow y[n] \tag{2}$$
This follows from the fact that both cascaded systems are LTI and order of LTI systems can be interchanged which follows from the fact that convolution operator is commutative and associative; i.e., $$h_1[n] \star h_2[n] \star x[n] = h_2[n] \star h_1[n] \star x[n] $$
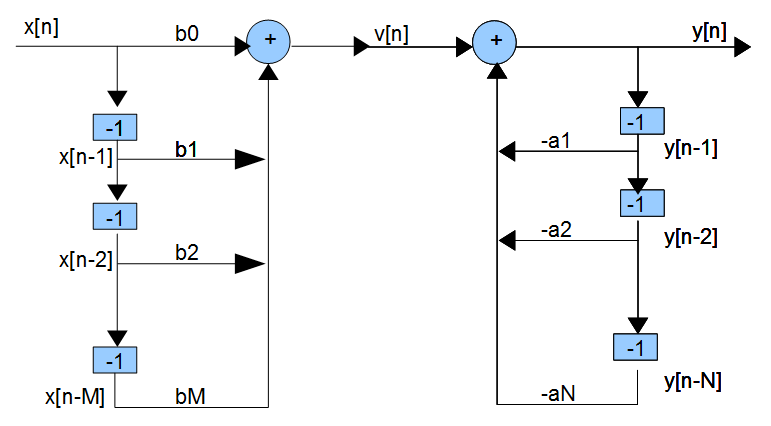
Now, the block diagram of (1) can be implemented with the following signal flow graph which yields a Direct-Form-I structure :
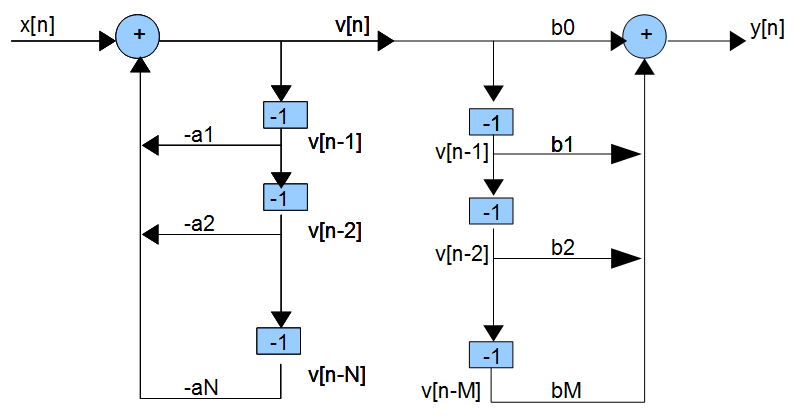
And, the block diagram of (2) can be implemented with the following signal flow graph which yields a Direct-Form-II structure:
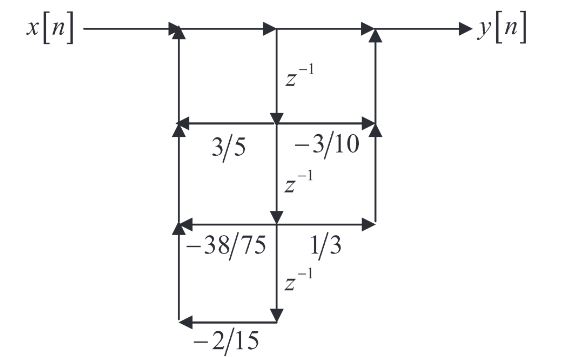
The intermediate memory (delays) are merged and the signal flow graph is simplified into the canonical direct-form II form finally as (assuming $N > M$)

From this last figure, it can be deduced that your LCCDE relating the input $x[n]$to the output $y[n]$ is :
$$ y[n] - \frac{3}{5} y[n-1] + \frac{38}{75} y[n-2] + \frac{2}{15} y[n-3] = x[n] - \frac{3}{10} x[n-1] + \frac{1}{3} x[n-2] $$