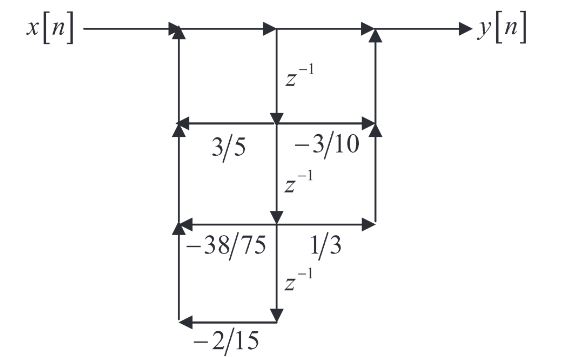
I am trying to solve for the difference equation of the following signal flow graph:
I am aware that direct form 2Direct Form II can be simplifiedconverted to direct form 1Direct Form I, which finding the difference equation directly is much easier. What I have come up with is defining v[n]an intermediate signal $v[n]$ as the top middle node. I get the following result,
$v[n]=x[n]+3/5*v[n-1]-38/75*v[n-2]-2/15*v[n-3]$
$y[n]=v[n]-3/10*v[n-1]+1/3*v[n-2]$$$\begin{align} v[n] &= x[n] + \tfrac{3}{5}v[n-1] - \tfrac{38}{75}v[n-2] - \tfrac{2}{15}v[n-3] \\ y[n] & =v[n]-\tfrac{3}{10}v[n-1]+\tfrac{1}{3}v[n-2] \\ \end{align}$$
As you can see, v[n]$v[n]$ is recursively dependent on itself. I know I could transform the two difference equations into their system functions and multiply together, find the total system function, and then transform back to their total difference equation. But for practice, I would like to know if their is another way to do this. I would like to somehow find the total difference equation without using their system functions.
Any advice would be appreciated! Thank you!