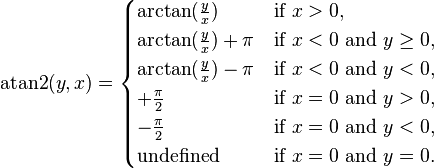Phase ambiguity in QAM modulation

Question

I'm currently studying a decision directed carrier recovery scheme. Basically a PLL try to estimate the phase error in the current received symbol, the phase detector compensate the incoming symbol's phase with the estimated one and then the result is multiplied my the complex conjugate of the decision taken. My notes say:

Another phenomenon that should be taken into account is the phase ambiguity of $n \pi/2$ that affects the decisions with conventional QAM constellations.

What is he talking about? I don't understand why he tells that there is phase ambiguity in the decision of a QAM symbol. Also citing Wikipedia:

Most QAM constellations also have $\pi/2$ phase symmetry

To me there is no phase ambiguity, in fact if I have a 4-QAM with 1 symbol in each quadrant I can just sample the I and Q branch after a matched filter and than perform a simple unambiguous atan like this:

Answer 1

Typically, phase ambiguity arises in the demodulation process when the carrier recovery is done via a loop that tracks the fourth harmonic of the received signal. So why can't the loop track the carrier frequency directly? Well, the received signal doesn't have a carrier signal -- it has a modulated carrier signal whose phase is going to change at every symbol interval which makes the phase untrackable by a typical loop -- and it is necessary to take the fourth power to eliminate the modulation and produce a signal with unchanging phase. As a result, the local oscillator (LO) in the receiver might be in any of four different phases differing by $\pi/2$, and the local reference signals to the mixers in the I and Q branches of the receiver might end up being not the expected \begin{align}\big(\cos(\omega t), -\sin(\omega t)\big)& & \text{LO and received signal are in phase}\end{align} as reference signals (note that the Q branch reference leads the I branch reference by $\pi/2$) but instead \begin{align}\big(\sin(\omega t),\cos(\omega t)\big)& & \text{LO lags received signal by }\pi/2\end{align} or \begin{align}\big(-\cos(\omega t), \sin(\omega t)\big)& & \text{LO lags received signal by }\pi\end{align} or \begin{align}\big( -\sin(\omega t),-\cos(\omega t)\big)& & \text{LO lags received signal by }3\pi/2.\end{align} The result is that instead of the I and Q bits appearing at their respective demodulator outputs (the receiver produces $(I,Q)$), the receiver might be producing $(\bar{Q},I)$, or $(\bar{I},Q)$, or $(\bar{Q},I)$ where the overbars denote bit complements. Differential demodulation is commonly used to eliminate the phase ambiguity.

Answer 2

My Digital Communication's theory is pretty rusty but here goes:

Consider the constellation diagram of a modulation scheme like the 4-QAM you proposed. Let's assume, without loss of generality, that our symbols are aligned with the x and y axis.

That is one symbol lies on the real (in-phase) axis in the positive half, one in the the negative half, and a matching pair on the quadrature axis. Consider what would happen if you rotated this by 90 degrees. Each symbol would appear to be a different one right? This is where the ambiguity comes in.

Phase ambiguity manifests as a rotation of the constellation diagram. We know the relative phase between received symbols when we demodulate, but we don't know which is the 'true' rotation of the constellation diagram because we don't know the actual phase of the incoming signal. One approach to getting around this is to use a training sequence of known data.