$G_0(e^{j\omega})$ and $H_0(e^{j\omega})$ are ideal low pass filters with passband $[0,\pi/2]$ and stopband $[\pi/2,\pi]$. $G_1(e^{j\omega})$ and $H_1(e^{j\omega})$ are ideal high pass filters that are complementary to the lowpass filters, i.e., their passbands coincide with the lowpass filters' stopbands and vice versa.
Note that shifting the frequency axis by $\pi$ transforms a lowpass filter to a highpass filter and vice versa. I.e., $H_0(e^{j(\omega-\pi)})$ is a highpass filter, and $H_1(e^{j(\omega-\pi)})$ is a lowpass filter. Consequently, $G_0(e^{j\omega})H_0(e^{j(\omega-\pi)})=0$ and $G_1(e^{j\omega})H_1(e^{j(\omega-\pi)})=0$. So the second term $(111b)$ corresponding to the aliased input spectrum vanishes. We're left with the first term, which is constant because we add an ideal lowpass spectrum and its complementary highpass spectrum.
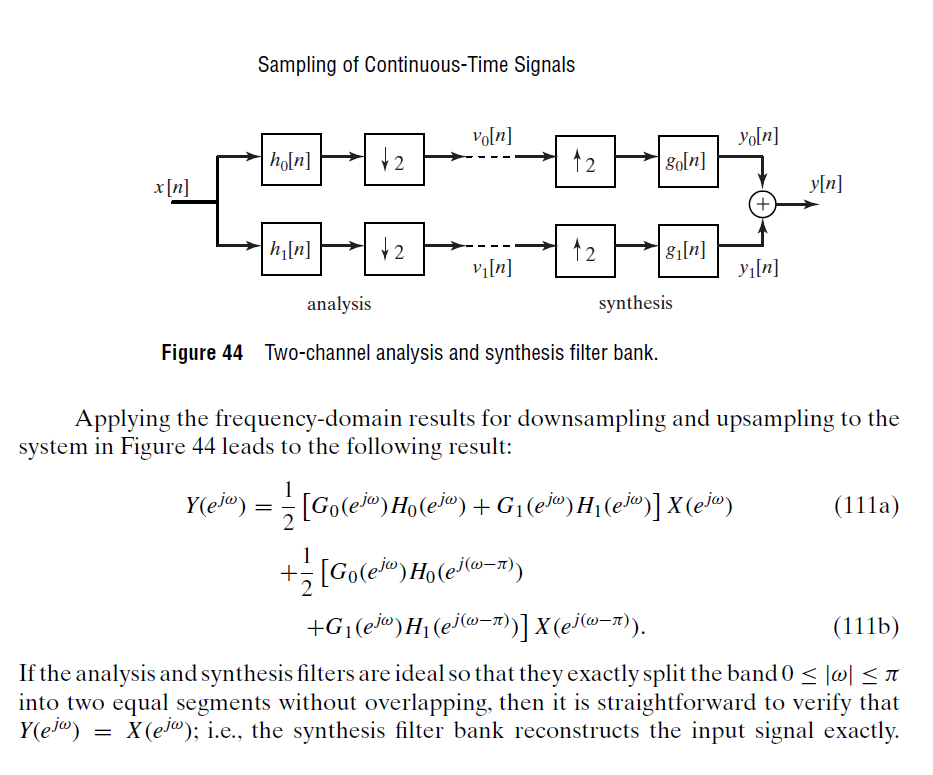 [from Discrete-time Signal Processing by Oppenheim and Schafer, 3rd ed.]
[from Discrete-time Signal Processing by Oppenheim and Schafer, 3rd ed.]