Excerpted from Jae S.Lim 2D signal and image processing ch.1, as an example of $2$-D circularly symmetric lowpass filter with a cutoff frequency of $\omega_c$ radians per sample, whose impulse response is given by:
$$h[n_1,n_2] = \frac{\omega_c}{2\pi \sqrt{n_1^2 + n_2^2} } J_1 \big( \omega_c \sqrt{n_1^2 + n_2^2} \big) $$
where $J_1$ is the Bessel function of the first kind and the first order...
Interested readers may consult the book for a derivation which is not untitive but nevertheless tractable; familiarity with Bessel functions is required but also provided as is.[Derivation is added.]
[Olli below]
At $n_1 = n_2 = 0$ the limiting value must be used:
$$h[0, 0] = \frac{\omega_c^2}{4\pi}$$
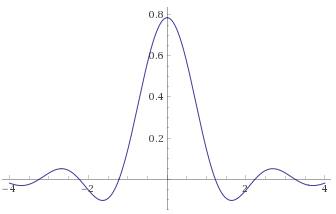
A slice through the middle of $h[n_1,n_2]$ with $\omega_c = \pi$:
Python source for 2-d filter kernel (you may want to apply a 2-d window function):
from scipy import special
import numpy as np
def circularLowpassKernel(omega_c, N): # omega = cutoff frequency in radians (pi is max), N = horizontal size of the kernel, also its vertical size.
with np.errstate(divide='ignore',invalid='ignore'):
kernel = np.fromfunction(lambda x, y: omega_c*special.j1(omega_c*np.sqrt((x - (N - 1)/2)**2 + (y - (N - 1)/2)**2))/(2*np.pi*np.sqrt((x - (N - 1)/2)**2 + (y - (N - 1)/2)**2)), [N, N])
if N % 2:
kernel[(N - 1)//2, (N - 1)//2] = omega_c**2/(4*np.pi)
return kernel
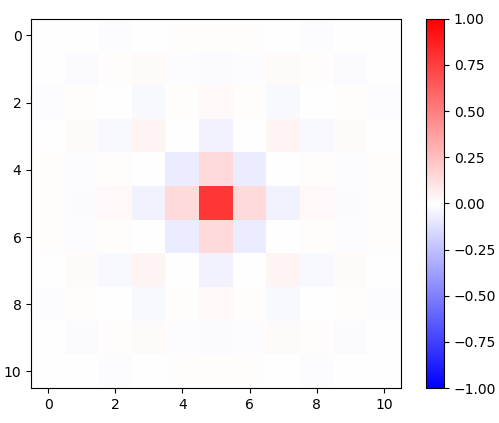
Example with $\omega_c = \pi$:
import matplotlib.pyplot as plt
kernelN = 11 # Horizontal size of the kernel, also its vertical size.
omega_c = np.pi # Cutoff frequency in radians <= pi
kernel = circularLowpassKernel(omega_c, kernelN)
plt.imshow(kernel, vmin=-1, vmax=1, cmap='bwr')
plt.colorbar()
plt.show()
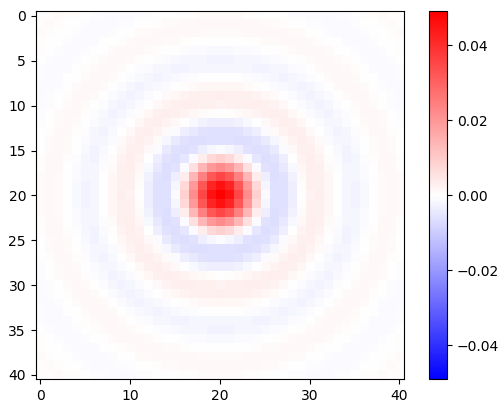
Example with $\omega_c = \pi/4$:
kernelN = 41 # Horizontal size of the kernel, also its vertical size.
omega_c = np.pi/4 # Cutoff frequency in radians <= pi
kernel = circularLowpassKernel(omega_c, kernelN)
plt.imshow(kernel, vmin=-np.max(kernel), vmax=np.max(kernel), cmap='bwr')
plt.colorbar()
plt.show()
[fat32 below]
Ok, for those who would benefit from a (not so intuitive) derivation, here I make a (n almost) verbatim copy of it from the same book.
First, lets simply write the 2D discrete-time inverse Fourier transform to define the impulse response as:
$$ h[n_1, n_2] = \frac{1}{(2\pi)^2} {\int \int}_{\omega_1^2+\omega_2^2< w_c^2} 1 \cdot e^{j(\omega_1 n_1 + \omega_2 n_2)} d\omega_1 d\omega_2 \tag{1} $$
Let's make a change of variables $\omega_1 = r \cos(\theta)$ and $\omega_2 = r \sin(\theta)$ (effectively write the integral in (1) in the cyclindrical (or polar) coordinates) :
$$ h[n_1, n_2] = \frac{1}{(2\pi)^2} \int_{r=0}^{\omega_c} \int_{\theta=a}^{a+2\pi} e^{j r (\cos(\theta) n_1 + \sin(\theta) n_2)} r ~ dr d\theta \tag{2} $$
Now, make a further change of variables $n_1 = n \cos(\phi)$ and $n_2 = n \sin(\phi)$, with $n = \sqrt{ n_1^2 + n_2^2 }$ and obtain
$$ h[n_1, n_2] = \frac{1}{(2\pi)^2} \int_{r=0}^{\omega_c} r ~dr \int_{\theta=a}^{a+2\pi} e^{j r n \big( \cos(\theta) \cos(\phi) + \sin(\theta) \sin(\phi) \big) } d\theta \tag{3} \\\\$$
which is now:
$$ h[n_1, n_2] = \frac{1}{(2\pi)^2} \int_{r=0}^{\omega_c} r ~dr \int_{\theta=a}^{a+2\pi} e^{j r n \cos(\theta -\phi) } d\theta \tag{4} \\\\$$
defining $f(r) = \int_{\theta=a}^{a+2\pi} e^{ j r n \cos(\theta-\phi) } d\theta $ , then we get:
$$ h[n_1, n_2] = \frac{1}{(2\pi)^2} \int_{r=0}^{\omega_c} r f(r) dr \tag{5} $$
Now, exploring $f(r)$ we can apply the Euler's identity :
$$ f(r) = \int_{\theta=a}^{a+2\pi} \cos(r.n.\cos(\theta-\phi)) d\theta + j \int_{\theta=a}^{a+2\pi} \sin(r.n.\cos(\theta-\phi) ) d\theta \tag{6} $$
And we can notice that the imaginary part is zero (can check an integral table for that) and with $a = \phi$ then $f(r)$ becomes
$$f(r) = \int_{\theta=0}^{2\pi} \cos(r.n.\cos(\theta) ) d\theta \tag{7}$$
Now the integral in (7) is recognized as the the Bessel function of order zero, kind one $J_0(x)$ which is given as:
$$ J_0(x) = \frac{1}{2\pi} \int_{\theta =0}^{2\pi} \cos( x \cos( \theta) ) d\theta \tag{8} $$
from (7) and (8) we see that $f(r) = 2\pi J_0(r n) $...
And the last identity is given as is:
$$ x J_1(x) |_a^b = \int_a^b x J_0(x) dx \tag{9}$$
Now putting the impulse response into the form
$$h[n_1,n_2] = \frac{1}{(2\pi)^2} \int_{r=0}^{\omega_c} r 2\pi J_0( r n) dr \tag{10}$$
applying (9) onto (10) with the recognition that $x = r n$ and $dr = dx/n$ and $n= \sqrt{n_1^2+n_2^2}$ yields the result:
$$\boxed{ h[n_1,n_2] = \frac{\omega_c}{2\pi \sqrt{n_1^2+n_2^2}} J_1( \omega_c \sqrt{n_1^2+n_2^2}) } \tag{11}$$
It should be added that the function describing the impulse response ($11$) is called the Jinc function (in analogy to the sinc function):
$$\mathrm{jinc}(x)=\frac{J_1(x)}{x}\tag{12}$$
Using ($12$), the impulse response ($11$) can be written as
$$h[n_1,n_2]=\frac{\omega_c^2}{2\pi}\,\textrm{jinc}(\omega_c\rho),\qquad \rho=\sqrt{n_1^2+n_2^2}\tag{13}$$



