6
$\begingroup$

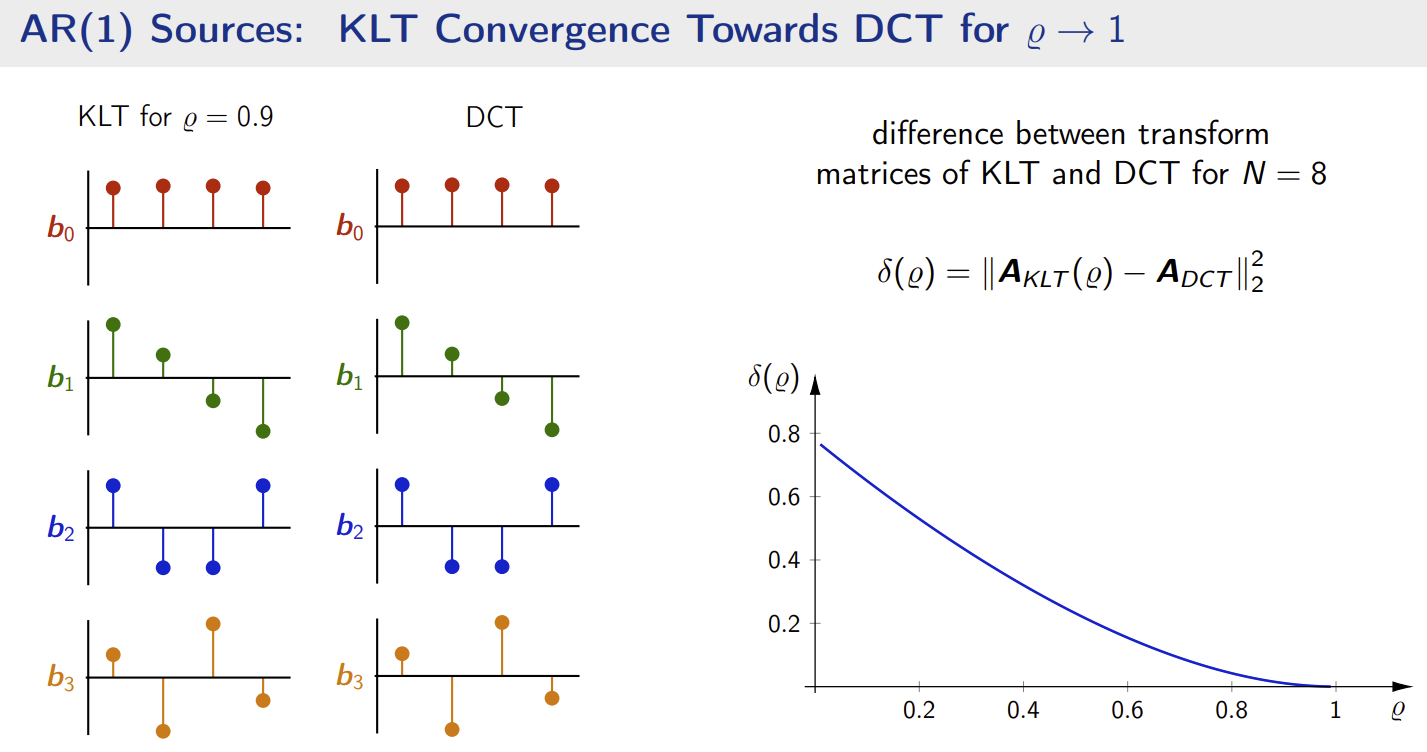
On the Wikipedia article about Discrete cosine transform it is said:

For strongly correlated Markov processes, the DCT can approach the compaction efficiency of the Karhunen-Loève transform (which is optimal in the decorrelation sense)

My question is: how can we show that the DCT approaches the efficiency of the Karhunen-Loève transform (also known as the Principal Components Analysis) and under which precise conditions?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Perhaps someone can digest this article to get an answer: Sanchez, V., Garcia, P., Peinado, A. M., Segura, J. C., & Rubio, A. J. (1995). Diagonalizing properties of the discrete cosine transforms. IEEE transactions on Signal Processing, 43(11), 2631-2641. $\endgroup$
    – Olli Niemitalo
    Commented Feb 26, 2023 at 6:37
  • $\begingroup$ Could you please review my answer? $\endgroup$
    – Royi
    Commented Jun 22, 2023 at 7:56

1 Answer 1

Reset to default
2
$\begingroup$

I can see that some papers refer to IEEE - N. Ahmed; T. Natarajan; K.R. Rao - Discrete Cosine Transform as a reference to the assertion that DCT is an approximation of the KLT.

Pay attention, to the assertion, it is a good approximation.

Another resource can be Heiko Schwarz - The Karhunen Loeve Transfrom:

enter image description here

The nice thing is that you can do it by yourself by generating data and apply the KLT and the DCT and compare the compression ratio to see it is well approximated.

Improve this answer
$\endgroup$
3
  • $\begingroup$ I don't have access to those papers. It would be nice to have a sketch of the proof / idea to show that there is a convergence. $\endgroup$
    – Weier
    Commented Jun 22, 2023 at 9:41
  • $\begingroup$ @Weier, Have you looked at the link which is open? $\endgroup$
    – Royi
    Commented Dec 8 at 19:04
  • $\begingroup$ Yes I've looked at it but it doesn't give much details. I was looking for a mathematical proof. $\endgroup$
    – Weier
    Commented Dec 11 at 9:43

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.