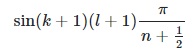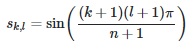An new arranging of the discrete Sine transforms

Question

Let $n$ be even and consider the non-normalized discrete Sine transform of type 5 which is

$$S=\left(\sin(k+1)(l+1)\frac{\pi}{n+\frac12}\right)_{k,l=0}^{n-1}$$

Let us denote $s_{-,l}$ by the $l^{th}$-column of $S$. It can be considered as a $n$-tuple in $\mathbb{R}^n$.

Q. I am looking for $n$-tuples $v=(v_0,\cdots,v_{n-1})$ in $\mathbb{R}^n$ satisfying the following conditions:

1- $v_j=\sin2(j+1)\frac{\pi}{n+\frac12}$ if $j$ is even.

2- The following are valid concerning inner products: $$\langle v , s_{-,l} \rangle=\left\{ \begin{array}{cl} 1 & l=0 \\ 0 & l\neq 0~,~ l \operatorname{is even} \end{array} \right.$$

Answer 1

Let's restate your problem as a linear equation

Let $U_{ee}$, $U_{eo}$, $U_{oe}$, $U_{oo}$ be the partition of the $S$ matrix in even-odd row-column index, similarly $v_e$ and $v_o$ are the partitions of $v$.

Restating your conditions in terms of matrices

1 - $v_e$ is fixed

2 - $v_e U_{ee} + v_o U_{oe} = I_1$, where $I_1$ denotes the first row of the identity matrix.

Placing all the constant terms on the right side of the condition 2, $v_o U_{oe} = (I_1 - v_e U_{ee})$, transposing we get a linear equation system in the standard form $U_{oe}^{T} v_o = (I_1 - v_e U_{ee})^T$

Proof that $U_{oe}$ has no pair of linearly dependent columns

Assuming

$s_{2i+1, 2j} = \sin\left( \frac{2\pi (2i+2)(2j+1)}{2n+1} \right)$

Two columns will be linearly dependent if, and only if, there are two integers $0 \le j_1, j_2 \le n/2-1$, for all integer $0 \le i \le n/2-1$

$$(2i+2)(2j_1 + 1) \equiv (2i+2)(2j_2 + 1) \operatorname{mod} (2n+1), $$

$$(2j_1 + 1) \equiv (2j_2 + 1) \operatorname{mod} (2n+1)$$

$$j_1 \equiv j_2 \operatorname{mod} (2n+1)$$

since both $j_1$ and $j_2$ are positive integers, smaller than $2n+1$, the condition can only be satisfied with $j_1 = j_2$, thus the matrix $U_{oe}$ is non-singular.

Answer 2

1.- for any other reader not familiar with DST type 5 or DST-V, I'd like to include the definition of Discrete Sine Transform and types.

Following a list with DST types 1 to 5, from

https://planetmath.org/discretesinetransform

1D DST types 1st to 8th

2.- Bob's assumption is correct. The question expression

actually means

and I'd like to add that the question expression for DST-V

is this

3.- DST Discrete Sine Transform and the counterpart DCT Discrete Cosine Transform are just partials of the DFT Discrete Fourier Transform.

Like Wolfram concisely explains here

https://mathworld.wolfram.com/DiscreteFourierTransform.html

and here

https://mathworld.wolfram.com/Leakage.html

Unless the signal or signal fragment is periodic in the watched interval, there's always (spectral) leakage.

4.- So, if the unknown input signal were all ones, satisfying the 2 conditions in the question would go the Bob answered.

5.- Yet it is up to x(:)*sin((k+1)*(L+1)*pi/(N+1)) not just the term sin((k+1)*(L+1)*pi/(N+1) to meet the non-leakage condition mentioned in point 2.

And since x is not supplied in the question in any shape or manner. there's no way to tell without knowing x .

5.- Is this a MATLAB question anyway?

I politely suggest this question to be moved to a Stack Overflow Maths page, or to for instance signal analysis or sampling tags in this same Signal Processing page

Thanks for reading.