0
$\begingroup$

Followup; setting Fs = bandWidth in accepted answer's code yields the "correct" frequency response of an LFM chirp: constant magnitude, parabolic phase. Setting it to anything else, including >> bandWidth, yields distortions, and magnitude converges to a square in the limit Fs / bandWidth -> inf.

Why's this the case? Shouldn't greater Fs be better - less aliasing, greater resolution, etc?

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ I think you are still confused about what the book presented and what increasing the sampling rate does to the spectrum (you get to see a wider bandwidth). There is no real distortion as you increase the sampling rate (as a matter of fact it's the opposite). You are simply seeing a wider bandwidth. I can answer this question if you'd like, but we're going back to fundamentals of sampling and the DFT, which is independent of LFM. $\endgroup$
    – Envidia
    Commented Sep 3, 2020 at 6:27
  • 1
    $\begingroup$ @Envidia Now that you say it, with the pictures floating just above... think I realize a few things. I've ran all my Python visuals within "perfect periods", i.e. signal bandwidth fit exactly between 0 and 0.5 - I suspected I'll see something else if I change it, but never got around to it. If it's as you say, then the 'square' is actually a flat line with the right 'zoom', which I think is what you were saying earlier. Good lead - I'll experiment. $\endgroup$
    – OverLordGoldDragon
    Commented Sep 3, 2020 at 6:45
  • $\begingroup$ Yup! That was exactly what I was saying. I'm sure you'll conclude it yourself after experimenting. $\endgroup$
    – Envidia
    Commented Sep 3, 2020 at 6:54
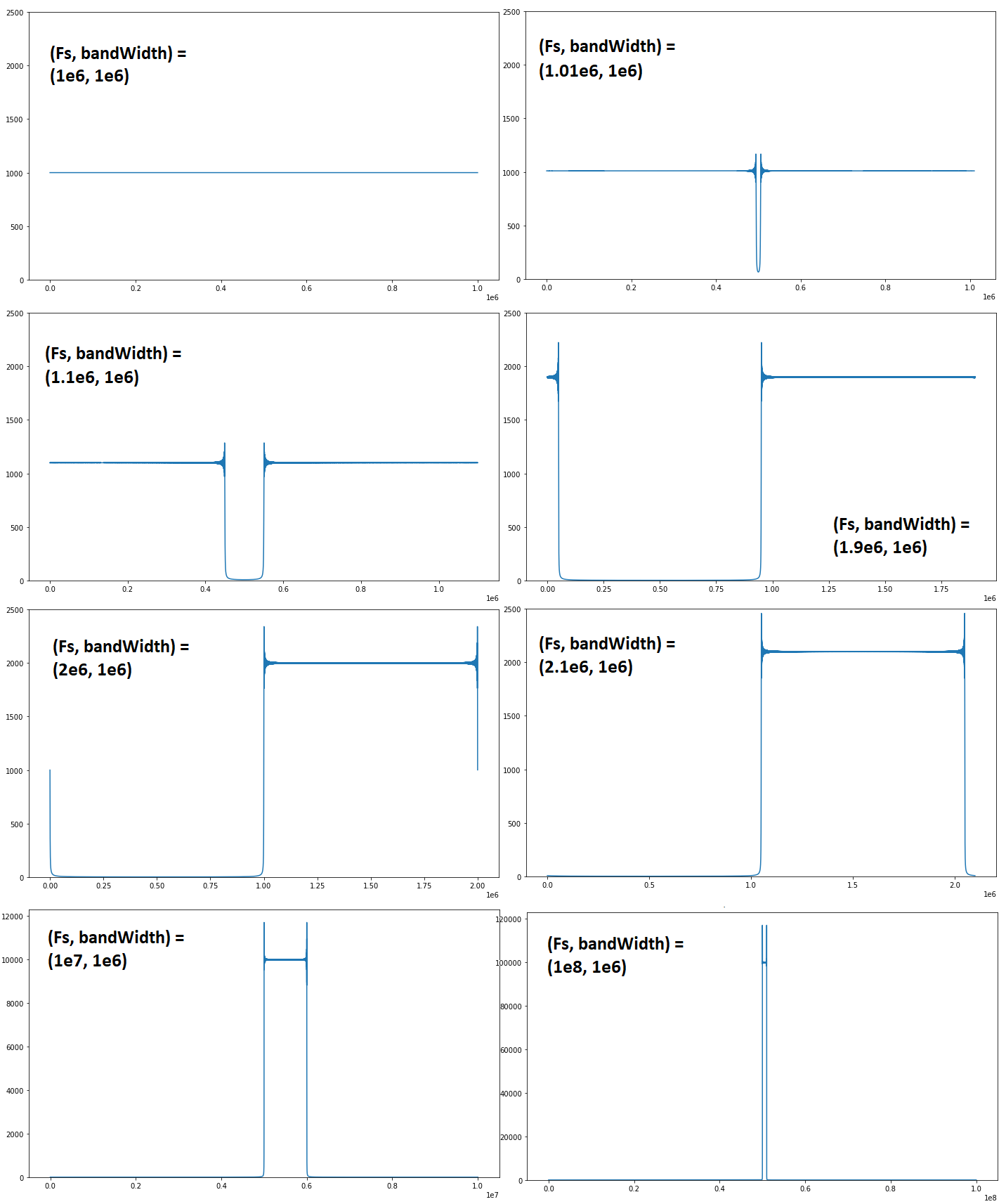
  • $\begingroup$ @Envidia Digging much further, I've yet to establish why a complex chirp's frequency response has perfectly constant magnitude when sampling at fs = bandWidth; this isn't about "zoom". Real chirp doesn't exhibit this either. I presume it's some convenient coincidence, but won't dig further into this - already chirped out. $\endgroup$
    – OverLordGoldDragon
    Commented Sep 8, 2020 at 12:41

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.