2
$\begingroup$

In attempting to answer this question by @Oliver here: What characterizies 'causality' for a finite FFT? I have considered the minimum requirement to avoid time domain aliasing in the Discrete Fourier Transform, or more generally any application where the frequency domain is sampled. Similar to sampling in time at at least twice the highest frequency to represent the spectrum without the effects of aliasing, I suggest using a time duration that is at least twice the response time of the underlying continuous time signal to represent the continuous time domain signal (in the DFT) without the effects of time aliasing. Or when the time domain process is restricted to known causal processes, the time duration is at least as long as the response time.

This is the equivalent to Nyquist's Sampling Theorem in the frequency domain; ultimately "sampling in frequency" such that the duration of the time domain waveform is greater than twice its response time.

I understand that the same theory would apply, but given that Shannon in his paper provides the Nyquist theorem in the time domain specifically has made me curious if this property may go by other formally named theorems in other domains?

To illustrate this graphically consider the drawing by RBJ below except replace the frequency axis with the time axis.

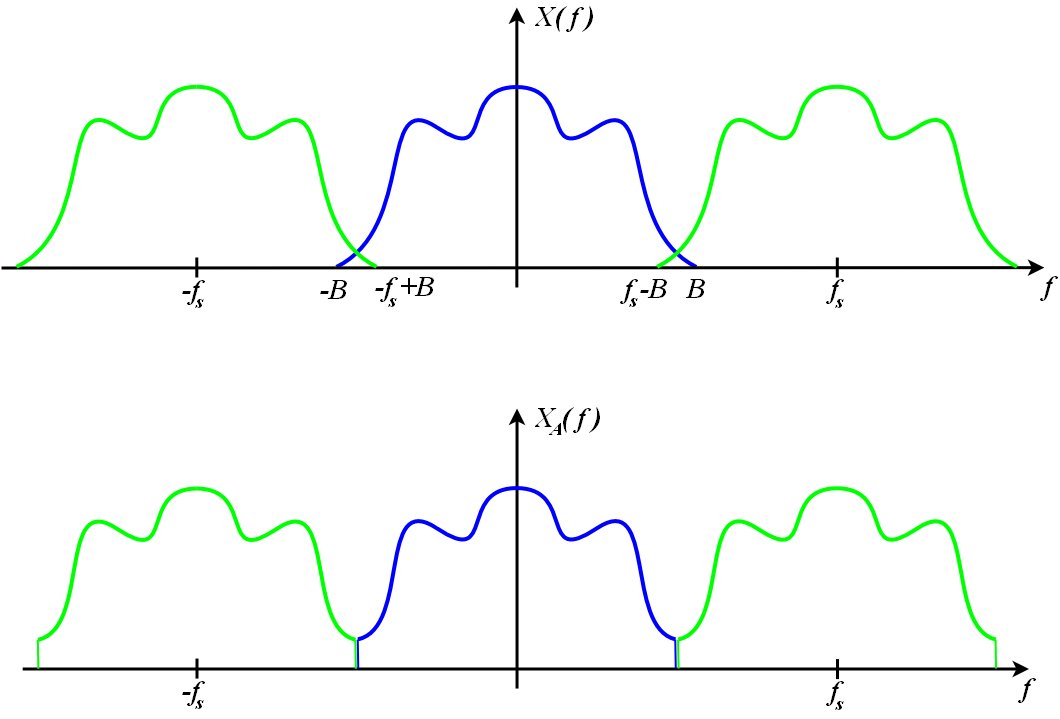enter image description here Public Domain, https://commons.wikimedia.org/w/index.php?curid=1065579

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ hay, i recognize that drawing. $\endgroup$
    – robert bristow-johnson
    Commented May 9, 2020 at 3:42
  • $\begingroup$ @robert oh look at that! It says user "Rbj". Nice drawing Robert, hopefully I did the attribution properly! $\endgroup$
    – Dan Boschen
    Commented May 9, 2020 at 11:16
  • 1
    $\begingroup$ Dan, if you read it, it says "No rights reserved". no need to attribute, you can take it and butcher the drawing, you can do anything with it you want. $\endgroup$
    – robert bristow-johnson
    Commented May 9, 2020 at 18:03

1 Answer 1

Reset to default
2
$\begingroup$

I'd say that this is not only "similar to a cross-domain equivalent to Nyquist's Sampling Theorem", but it simply is the sampling theorem. The sampling theorem does not specify the domains of the signals involved; it is rather a mathematical condition that a function of a continuous variable needs to satisfy such that it is perfectly represented by equidistant samples. It is irrelevant if the independent variable of that continuous function is time, frequency, space or anything else.

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ I thought that too (and that is applicable to all operations in either domain between time and frequency) but the original proof very clearly specifies the time domain and gives no mention to what I think would otherwise be obvious that it applies in either domain: web.archive.org/web/20100208112344/http://www.stanford.edu/… "Theorem 1: If a function contains no frequencies higher than cps, it is completely determined by giving its ordinates at a series of points spaced 1/2 seconds apart." $\endgroup$
    – Dan Boschen
    Commented May 9, 2020 at 11:09
  • $\begingroup$ Hence my question as I thought perhaps given this predominant application to the time domain the same theorem may formally occur in other forms applicable to the frequency domain. $\endgroup$
    – Dan Boschen
    Commented May 9, 2020 at 11:12
  • 1
    $\begingroup$ not that Matt needs the boost in the rep, but +1 from me for saying it as it really is. $\endgroup$
    – robert bristow-johnson
    Commented May 9, 2020 at 18:04
  • $\begingroup$ @DanBoschen: The proof is indeed given for a function of time, but nowhere does the proof rely on this fact. It is more that the application asked for sampling a function of time, hence this specialization. But the result is absolutely general, no matter if you sample in time, frequency, or space (or anything else). $\endgroup$
    – Matt L.
    Commented May 9, 2020 at 18:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.