I am new to signal processing and have been trying to understand the terminology. I came across this statement: "Assuming the signal modulation $\mathfrak{M}$ has an alphabet A of M symbols and the symbol $A_{m}$ having the equal probability to be transmitted". What is $A_{m}$ exactly here or what kind of array will it be? Can anyone help me understand this with any modulation example?
2 Answers
A symbol is a symbolic representation of a baseband signal in digital communication. Imagine you have 2 bits with equal probability 0 and 1. For 0 you transmit $s_0(t)=+A$ for $0 \le t \le T$. For 1 you transmit $s_1(t)=-A$ for $0 \le t \le T$. You are basically varying the amplitudes of a basic vector signal which is rectangle in this case $r(t) = A$ for $0 \le t \le T$. Here $s_0(t) = 1 \times r(t) $ and $s_1(t)=-1 \times r(t)$. The 'symbols' -1 and +1 are the scalars which multiply the fundamental signal. $A_m = \pm 1$.
If you vary phase instead of amplitude, you get schemes like BPSK(technically still AM), QPSK,8-PSK,...,M-PSK in general. For QPSK your inphase and quadrature bits both decide the phase offset. $x_{qpsk}(t) = (-1)^b_I(1/\sqrt{2})\cos(\omega_c t)-(-1)^b_Q(1/\sqrt{2})\sin(\omega_c t)$ which results in baseband equivalent phase offset of $i\times \pi/4$ where $i \in \{0,1,2,3\}$. $M=4$.$A_m = \pm 1 \pm j$.
For QAM, you vary both amplitude and phase, resulting in symbols which represent both phase as well as amplitude change. Example 16-QAM where $M=16$.
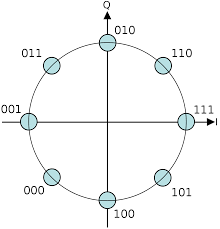
Below is the constellation diagram of 8 QPSK, The circles you see below are modulation symbols. The alphabet A here is the set of all these 8 possible symbols that are equally likely to be transmitted. Each symbol represents a bit pattern as you see in the diagram. each time a symbol is transmitted that bit pattern is essentially transmitted