I am trying to implement convolution using polyphase structure but, how to split input samples among different filter banks is my major doubt. Please explain the same with example?
$\begingroup$
$\endgroup$
5
-
$\begingroup$ Why do you want to use polyphase? It's only useful for specific applications and the best implementation depends on which application it is $\endgroup$– HilmarCommented Jan 17, 2020 at 8:36
-
$\begingroup$ @Hilmar it's for a real-time narrow band filter. Already implemented other efficient methods but, it's been decided polyphase would be the best bet. How do I convolve using polyphase structure?, I already have my filter coefficients separated. $\endgroup$– NagashreeCommented Jan 17, 2020 at 9:42
-
$\begingroup$ yeah, but the polyphase decomposition type you use depends on why you're doing a polyphase implementation, so: we're going in circles. Please tell us why you're doing this filter in polyphase. $\endgroup$– Marcus MüllerCommented Jan 17, 2020 at 10:03
-
$\begingroup$ @MarcusMüller I am doing this filter in polyphase for parallel processing and to have a smaller buffer requirement. Mainly I want to achieve continuous filtering and not hold any input sample. $\endgroup$– NagashreeCommented Jan 17, 2020 at 10:14
-
$\begingroup$ ee.ic.ac.uk/hp/staff/dmb/courses/DSPDF/01200_Polyphase.pdf: this is the pdf I went through before starting my implementation. $\endgroup$– NagashreeCommented Jan 17, 2020 at 10:21
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
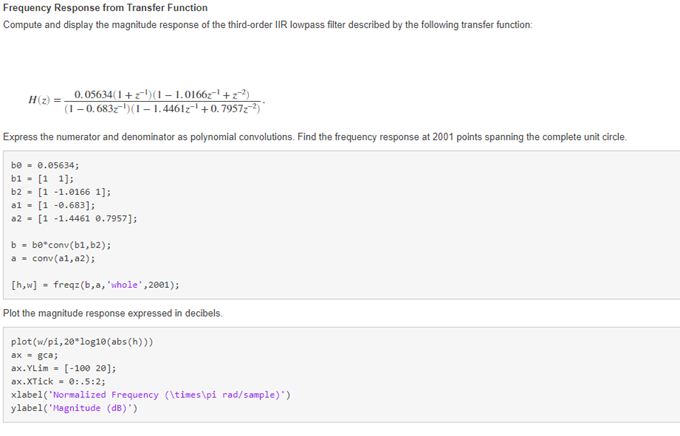
I am not quite sure, if i understand you question properly, it would be better if you ask with detail and maybe with a figure, nevertheless if you want convolution of co-efficents of the transfer function then below might be of help. :
and with regard to splitting.. you can easily do it with something like this :
x(1:2:end) = cos(2*pi*f*t(1:2:end));
x(2:2:end) = cos(2*pi*f*t(2:2:end));
-
$\begingroup$ I am looking for a way to convolve using polyphase structure. The design of the filter is in the following link: ee.ic.ac.uk/hp/staff/dmb/courses/DSPDF/01200_Polyphase.pdf But, I am not sure how the inputs are going into the downsampler and upsampler. Generally filter is designed with upsampler first and then the downsampler but, the link above says opposite. $\endgroup$ Commented Jan 17, 2020 at 11:43