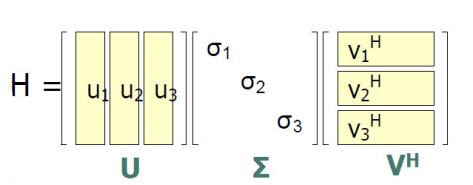
Why can we know the best precoding direction after we do the SVD to the channel?for example
$\mathbf y=\mathbf H\mathbf s+\mathbf n$,now we do the SVD to the $\mathbf H$
If the $\sigma _1$ is the biggest singular value,after we normalize $V_1$,why can we say the $V_1$ is the best precoding direction?Is there any theorem can prove this?