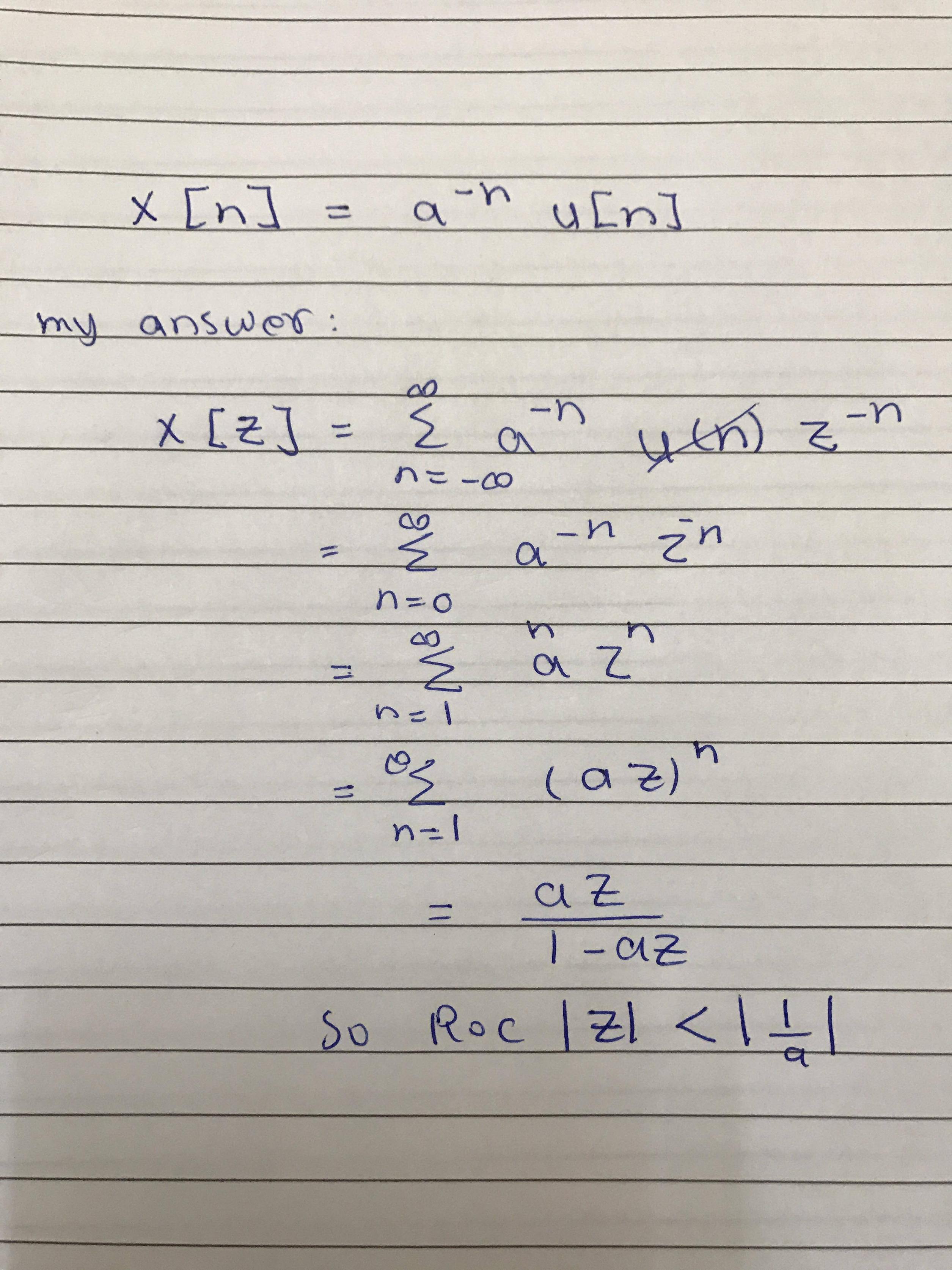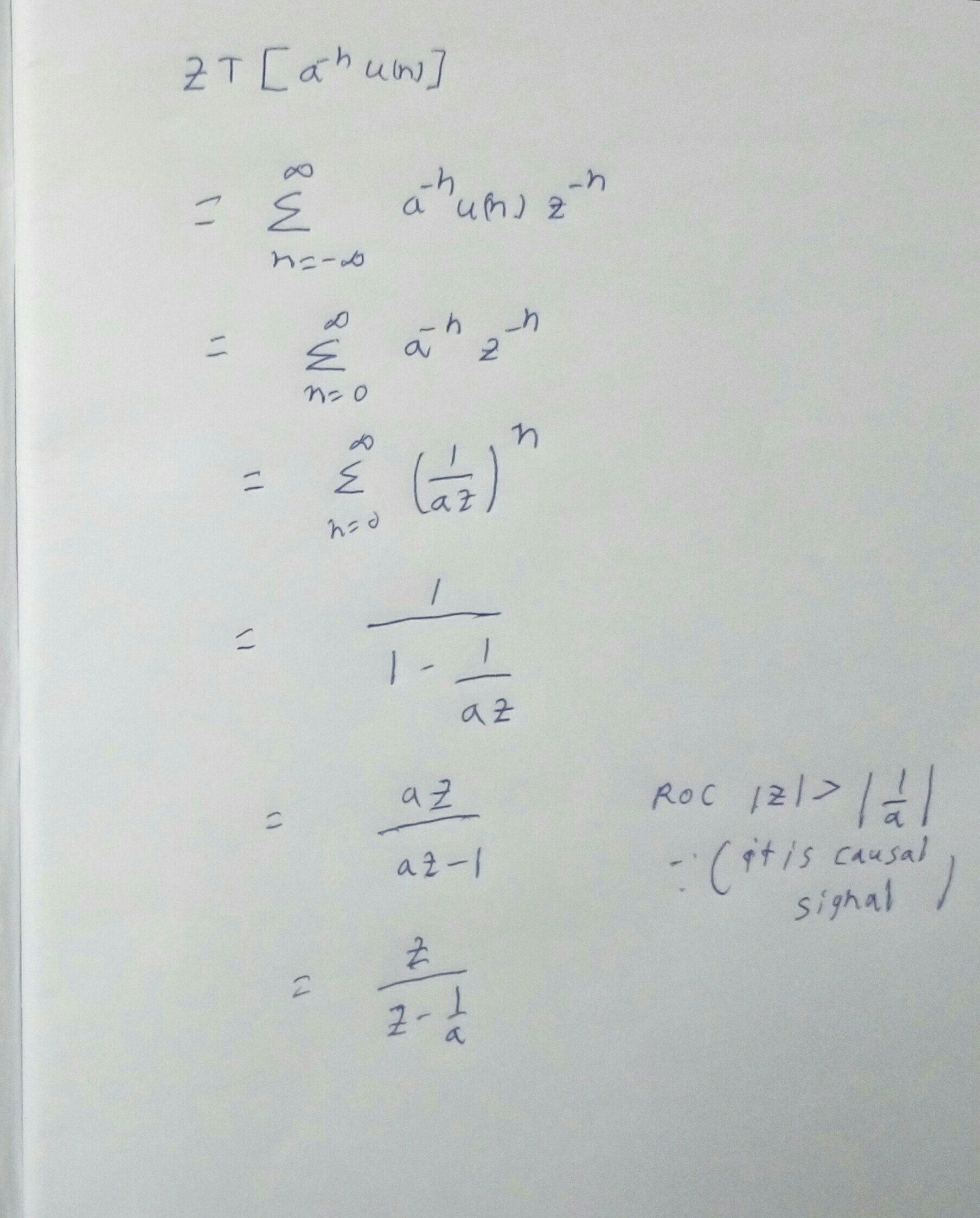I need to find the $\mathcal Z$-transform of $x(n)=a^{-n} u(n)$. Assume, $a$ is a positive constant , but the power of $a$ is negative.
Looking at the transform table, I found that $\mathcal Z$-transform for $x(n)=a^{-n} u(n)$ is not there, so I tried to found it by on my own, but I am not sure if my answer is right, please can anyone check it and correct me, in case?
Doing some calculations is appreciated. However, it is preferred that you write them in the question because a picture can vanish more easily. Do not hesitate to edit the math.
To help you with an hint in checking your answer, remember that:
$$ a^{-n} = \left(\frac{1}{a}\right)^n$$
a standard trick to play with known tables.
The $X(z)$ calculated is correct but there are some mistakes in the steps and ROC is wrong.
$X[z]$ is wrong representation of $\mathcal Z$-Transform since it is not a discrete sequence but rather a continuous function of $z$, where $z$ is a complex variable in argand plane ($z$-plane).
Limit of $\sum$ changes from $n=0$ to $\infty$ to $n=1$ to $\infty$. Why? That's not right.
$a^{-n}$ and $z^{-n}$ changes to $a^{n}$ and $z^{n}$ inside $\sum$. Not correct.
The ROC is the region on $z$-plane where the $\mathcal Z$ transform summation becomes finite in absolute sense. For any Causal Sequence like yours ($a^{-n}u[n]$), the ROC is complete region extending to $\infty$ from the largest pole of $\mathcal Z$-transform which is $\frac{1}{a}$ in your case. Basically, ROC will be $|az| > 1$ . This is easy to see from the $\mathcal Z$-Transform expression itself:
$X(z) = \sum_{n=0}^{\infty} (az)^{-n}$
Consider a Discrete time signal and analyze its transformation into DTFT, Z-transform. With examples represent various ROC for any signal or sequence
No you have done a small mistake how a^-n became a^n? So it is like this the ROC is right sided
