In engineering it is well known that we abuse the notation of a function $f: A \to B$ with the image of that function $f(t) = \left\{y \in B\mid f(t) = y, t \in A\right\}$
When we say: let $f(t) = \sin(\omega t)$ be a signal, do we mean a function or the set of output from that function i.e. is signal a function or the output?
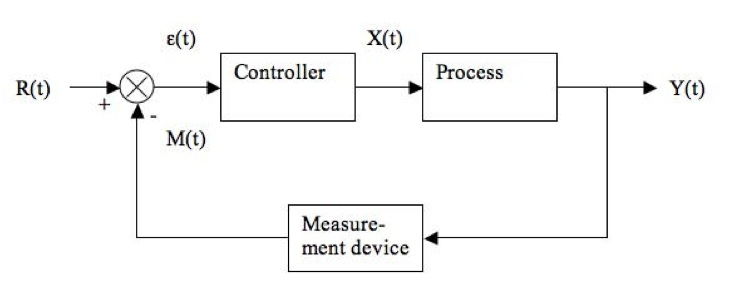
Similarly, looking at the diagram below, would one say that $\epsilon(t)$ is a set of inputs (i.e. scalars) going into the controller, or a function?