Can someone let me know linearity, time-invariance, causality, memory characteristic of the system?

Question

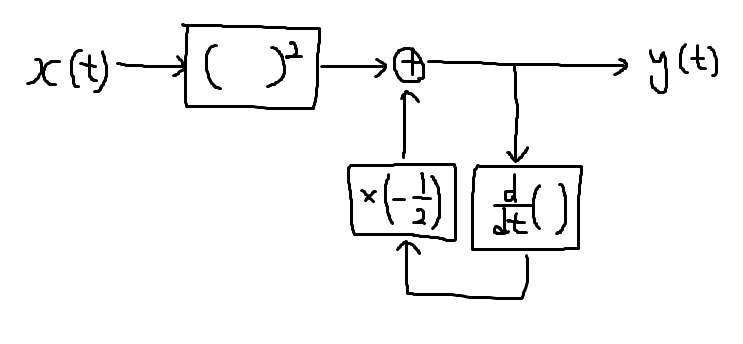
The input/output system is $$ \frac{dy(t)}{dt}+2y(t)=2x^2(t) $$

---

I want to know this is linear? causal? time-invariant? memoryless?

According to solution, the answer is 'Linear if zero initial conditions, causal, time-invariant, with memory'.

---

My trial:

$$y(t)=x^2(t)-\frac{1}{2}\frac{dy(t)}{dt}$$

Sorry for my poor drawing.

---

1. for input value: $x_1(t)=2x(t)$, \begin{align} y_1(t)&=\left(2x(t)\right)^2+\left(-\frac12\right)\frac{d}{dt}\left(y(t)\right)\\ &=4x^2(t)-\frac12\frac{dy(t)}{dt}\\ 2y(t)&=2x^2(t)-\frac{dy(t)}{dt}\\ \therefore y_1(t) &\ne 2y(t) \end{align}

   $\because$ not satisfying Homogeneity, Non-Linear.

---

2. The system doesn't have $x(t+k)$. (It means $y(t)$ is not made by $x(t+k)$)

$\therefore$ The system is causal because

---

3. for input value: $x_1(t)=x(t-t_0)$, \begin{align} y_1(t)&=x^2(t-t_0)-\frac{1}{2}\frac{dy(t)}{dt}\\ y(t-t_0)&=x^2(t-t_0)-\frac{1}{2}\frac{dy(t-t_0)}{dt}\\ \therefore y_1(t) &\ne y(t-t_0) \end{align}

   $\therefore$ time-varying.

4. The system doesn't have any $\displaystyle\int$.

   i.e.) $\displaystyle\int x(\tau) d\tau$.

   $\therefore$ memoryless

---

What is the real solution?

Answer 1

There's an error in your 3rd section (linearity) - you replace $y(t)$ with $y_1(t)$ on the left side of the equation but not on the right side. You should have $$y_1(t)=x^2(t-t_0)-\frac{1}{2}\frac{dy_1(t)}{dt}$$. Now you see that $y_1(t)=y(t-t_0)$.

As for memoryless - start with your initial equation and integrate both sides. Now you have $$ \int y(t)dt=\int x^2(t)dt-\frac{1}{2} y(t)dt $$

and rearranging gives $$ y(t) = 2 \int x^2(t)dt-2 \int y(t)dt $$ Does that help?