Performing STFT after Butterworth Filter Seems Lower in Resolution

Question

I have a signal recorded at 2MHz sampling rate. Before any decimation might be required, I first looked for peaks in my periodic recorded signal using STFT/spectrogram. From here, I can clearly see some peaks. Using PSD I could find that the frequencies were not prominent after ~500kHz so I apply the Butterworth filter to filter out frequencies higher than this. Also my antenna records from ~20kHz so frequencies below this are also filtered out.

The sample.wav file can be downloaded here: https://www.dropbox.com/s/hcwrtuzqbipatri/sample.wav?dl=0

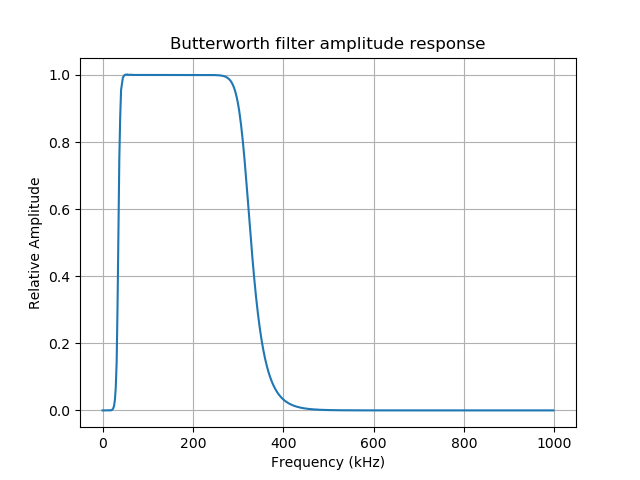
The filter amplitude response looks as follows:

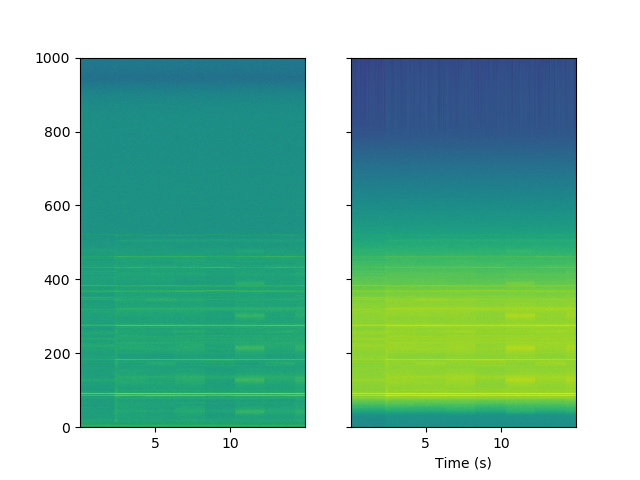
However, comparing the spectrograms for the original and bandpass filtered signals look fairly different and I can't seem to understand why. The original signal is on the left and the butterworth filtered signal is on the right. The y-axis scale is Frequency (kHz).

The code used for the filter can be viewed below:

import numpy as np
import os
import scipy.io.wavfile
from scipy import signal
# import librosa
import matplotlib.pyplot as plt
from sklearn.decomposition import PCA

fs, x = scipy.io.wavfile.read('sample.wav') # fs is 2MHz (2000000)
# Convert to mono by avg I (left) and Q (right) channels
x = np.mean(x, axis=1)
x = np.asarray(x, dtype='float')
# Once the recording is in memory, we normalise it to +1/-1
# x /= np.max(np.abs(x)) ## This is sensitive to outliers and rescaling is not consistent
x /= x.std()

t_tot = 1 / fs*x.shape[0]
t = np.linspace(0, t_tot, x.shape[0])
tmin = np.min(t)
tmax = np.max(t)
N = len(x)

f_l, f_u = 20000, 500000  # Band from 20kHz to 500kHz
wp = np.array([f_l, f_u])*2/fs  # normalized pass band frequnecies
ws = np.array([0.8*f_l, 1.2*f_u])*2/fs  # normalized stop band frequencies
b, a = signal.iirdesign(wp, ws, gpass=60, gstop=80, ftype="butter", analog=False)

# plot filter response:
w, h = signal.freqz(b, a, whole=False)
ff_w = w*fs/(2*np.pi)
fg, ax = plt.subplots()
ax.set_title('Butterworth filter amplitude response')
ax.plot(ff_w/1000, np.abs(h))
ax.set_ylabel('Relative Amplitude')
ax.grid(True)
ax.set_xlabel('Frequency (kHz)')
fg.canvas.draw()
# do the filtering:
zi = signal.lfilter_zi(b, a)*x[0]
x1, _ = signal.lfilter(b, a, x, zi=zi)
# calculate the avarage:
avg = np.mean(x1**2)
print("RMS values is %g" % avg)
plt.show()

fig, ax = plt.subplots(1, 2, sharex=True, sharey=True)
f, t, Sxx = signal.spectrogram(x, fs=fs, window='hann', nperseg=8192, scaling='spectrum')
ax[0].pcolormesh(t, f / 1000, 10 * np.log10(Sxx), shading='gouraud')

f_1, t_1, Sxx_1 = signal.spectrogram(x1, fs=fs, window='hann', nperseg=8192, scaling='spectrum')
ax[1].pcolormesh(t_1, f_1 / 1000, 10 * np.log10(Sxx_1), shading='gouraud')
# plt.set_title(f'nperseg={str(i)}')
plt.xlabel('Time (s)')
plt.ylabel('')
plt.show()

Answer 1

Color norm is the problem. By default, colors are mapped from im.min() to im.max(), so a single high-valued pixel will make the entire spectrogram appear darker. I.e. it's sensitive to outliers (and why I discouraged x /= x.max()).

When plotting images for comparison, we should always specify a shared color range. To ensure nothing's lost, we can take max of all images - but that might lose detail, so we might do like 0.5 * im.max() until satisfied. The code I provided in comments accomplishes this and yields

In your case you took log10 hence the outlier is actually im.min(), and clearly on left graph, the values near top are smaller than near bottom, so after decimation the color range doesn't need to account for these and more richly maps out remaining variation (greater color dynamic range for lesser input dynamic range).

Proper decimation will not alter time-frequency behavior except near "transition point" in frequency domain (~300 Hz in your graph), so the spectrogram should remain identical outside that region. (You aren't actually decimating but the filter closely resembles one of decimation due to a "flat top", i.e. bins aren't scaled relative to each other as in e.g. Gaussian)