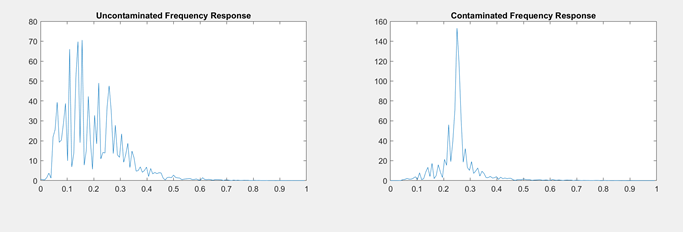
 I have the frequency response of two signals shown above. One is uncontaminated, and the other is the same signal contaminated by a multiplicative distortion with two poles and two zeros. I need to find/estimate the transfer function values of the contaminating filter in order to equalize the contamination. How do I accomplish this in MATLAB? Thanks.
I have the frequency response of two signals shown above. One is uncontaminated, and the other is the same signal contaminated by a multiplicative distortion with two poles and two zeros. I need to find/estimate the transfer function values of the contaminating filter in order to equalize the contamination. How do I accomplish this in MATLAB? Thanks.
-
1$\begingroup$ Multiplicative in Frequency Domain or Time Domain? I guess in frequency but it is better to explicitly say it. As then it is not a multiplicative distortion but a Filter. $\endgroup$– RoyiCommented Dec 6, 2020 at 5:38
-
1$\begingroup$ Can you share a CSV file with your measurements? $\endgroup$– RoyiCommented Dec 7, 2020 at 5:31
1 Answer
Basically the transfer function is given by (Continuous time case):
$$ H \left( j \omega \right) = K \frac{ \left( j \omega - {q}_{1} \right) \left( j \omega - {q}_{2} \right) }{ \left( j \omega - {p}_{1} \right) \left( j \omega - {p}_{2} \right) } $$
Now, given your frequency data sampled an $ N $ points $ {\left\{ {\omega}_{i} \right\}}_{i = 1}^{N} $ we need to use the discrete-time form of the transfer function over the Z domain (Z Transform).
Hence the model becomes:
$$ H \left( z \right) {\mid}_{z = {e}^{j \omega}} = K \frac{ \left( z - {q}_{1} \right) \left( z - {q}_{2} \right) }{ \left( z - {p}_{1} \right) \left( z - {p}_{2} \right) }{\Bigg|}_{z = {e}^{j \omega}} = K \frac{ \left( {e}^{j \omega} - {q}_{1} \right) \left( {e}^{j \omega} - {q}_{2} \right) }{ \left( {e}^{j \omega} - {p}_{1} \right) \left( {e}^{j \omega} - {p}_{2} \right) } $$
you may set a system of $ N $ complex equations and use non linear solver to find the 5 parameters ($ K $, $ {q}_{1} $, $ {q}_{2} $, $ {p}_{1} $, $ {p}_{2} $) you need.
In case the measurements are noisy you may use Least Squares.
Remark
The above solves the problem from the data of @jmcd in frequency domain. In practice, using the data domain one could use simpler System Identification (See System Identification: An Overview) methods to get the equivalent ARMA model.
Regarding Frequency Domain based methods for System Identification have a look at Rik Pintelon - System Identification: A Frequency Domain Approach.
-
$\begingroup$ is this problem in continuous time or discrete time? $s$ domain or $z$ domain? $\endgroup$ Commented Dec 6, 2020 at 19:33
-
$\begingroup$ You are right, I should have used a domain syntax to be clearer. $\endgroup$– RoyiCommented Dec 6, 2020 at 20:47
-
$\begingroup$ but Royi, the question is still there. it's pretty clear to me that you are suggesting an answer (i think it's sorta a partial answer because no final solution is given, even with the Least Squares, but that's fine) that is in the continuous-time domain ($H(s)\Big|_{s=j\omega}$). perhaps the transfer function the OP is looking for is in the discrete-time domain $H(z)\Big|_{z=e^{j\omega T}}$. that will be a slightly different looking frequency response and the LS algorithm might come up with different poles and zeros (given the mapping $z=e^{sT}$). $\endgroup$ Commented Dec 7, 2020 at 3:43
-
$\begingroup$ may i modify your answer to cover both bases? $\endgroup$ Commented Dec 7, 2020 at 3:45
-
