Ideal reconstruction after down sampling

Question

The signal $x_a(t) = \cos(2\pi450t)$ is sampled.

F = 450
Fs = 1000 Hz
f = F/Fs = 450/1000   // Sampling theorem is fulfilled
x(n) = cos(2*pi*(450/1000))

The signal is then down sampled with a factor 3.

fNew = f*3 = 450*3/1000 = 1.35
xNew(n) = cos(2*pi*1.35)

Now the signal is prepared. How to make an ideal reconstruction using 1000Hz?

Answer 1

If you take your input signal and downsample it by a factor of 3 your original frequency of 450 Hz will be aliased. But since you single contains a single frequency it can be reconstructed.

For example (in MATLAB), let's generate 1024 samples of your signal:

Fs = 1000;
t = (0:1023)/Fs;
xa = cos(2*pi*450*t);
figure; pwelch(xa,[],[],[],Fs);

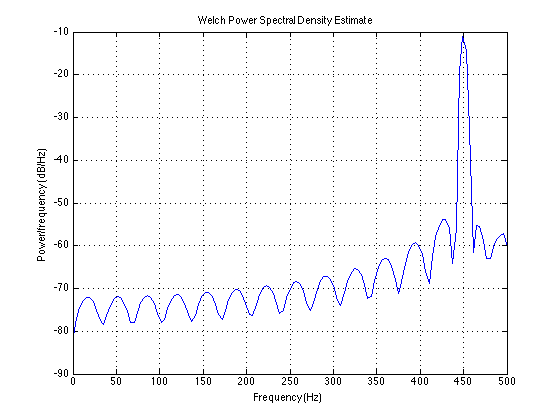
Now if you down sample your signal you notice that the frequency has changed.

xad = xa(1:3:end);
figure; pwelch(xad,[],[],[],Fs/3)

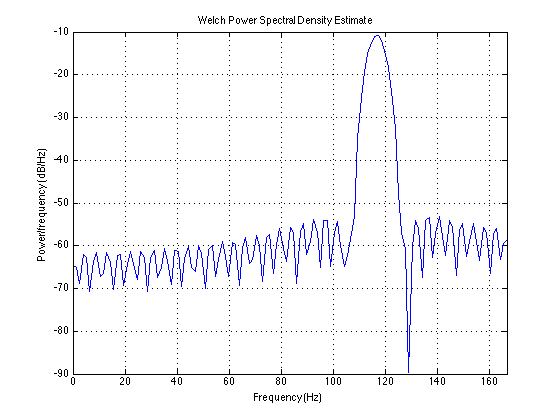
And if you try to interpolate the signal back to the original sampling frequency, you can see that there is some kind of "ambiguity":

xadu = upsample(xad,3);
figure; pwelch(xadu,[],[],[],Fs);

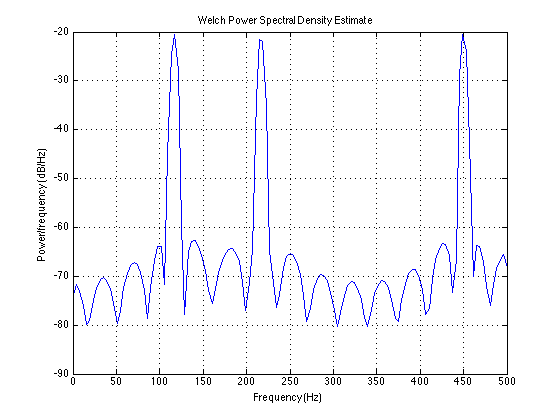
Also note that the amplitude is lower (by a factor of 3 actually).
All there is left is to filter the signal using a high-pass filter:

h = fir1(32,350/500,'high');
xaduf = filter(3*h,1,xadu);
xar = xaduf(37:end);

Answer 2

The signal can not be reconstructed because in the new sampled signal the sampling theorem is violated. Aliasing would occur and the signal reconstructed would be a lower frequency than the original signal.