In the book "The control handbook. Volume 1 " by Levine, the author shows that the transfer function:
[![enter image description here][1]][1]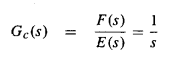
can be aproximated and discretized in the transfer function:
[![enter image description here][2]][2]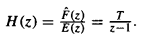
using the forwar euler integral aproximation. In this way Levine shows that if i have a transfer function $G(s)=\frac{1}{s}$ i can simply substitute $s=\frac{z-1}{T}$ to apply the Forward Euler aproximation. At this point the author doesn't show how to generalize this particular case to a generic transfer function $H(s)$. Are you able to show me ? [1]: https://i.sstatic.net/H9zB6.png [2]: https://i.sstatic.net/SuGJ3.png
