Sorry for the vague question (as I'm not even quite sure what I want to do is possible), just asking for some general direction to take my research.
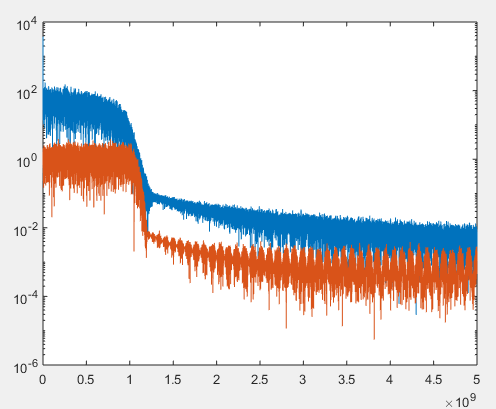
For a brief description, my signal resembles exponentially distributed noise, bandlimited, with a triangle shaped spectrum around the baseband. I'm looking to remove additive white Gaussian noise within the same frequency band as the signal, ideally without affecting the signal bandwidth.
Below are some simulated examples of a section of my signal and noise:
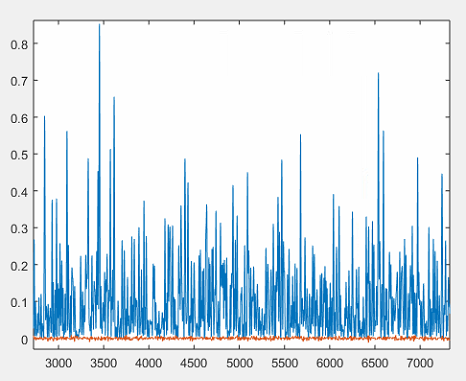
and their bandwidth
So the question is whether I can, somehow, attempt to improve the SNR, given that they share the same frequency band, and my signal is not known beforehand (though it differs from the noise in distribution and spectral shape).
EDIT Averaging successive acquisitions is not an option, as what we want to measure is a local time delay of the blue trace. As such, just averaging would interfere with the measured delay.
EDIT 2 I see people commenting that the SNR is fairly high in this case, just want to clarify: This is the current use case and we are currently happy with the performance. In the case of our system, without going too much into irrelevant details, if we increase the spatial resolution the SNR will deteriorate (up to 10 or 20dB), so maybe the representation isn't accurate of what I was trying to convey. I will post new figures as soon as I am back in the office, or if I get an opportunity tomorrow of a noisier case.