What is the audible level for digital audio dB units?

Question

What is the audible level for digital audio dB units?

I was looking at a soundwave from an mp3 file. I am using the "Sonic Visualizer" app available on Linux. The amplitude is between ~ -0.1,0.1 in my file. I have already learned the amplitude is on [-1,1].

The soundwave is between ~ -40,-10 when I convert the soundwave to a dB y-scale. I have that dB conversion formula.

I read online that the human ear starts to hear near -10dB.

What does that 0-ish threshold correspond to in digital audio? Why are the dB reported in these audibility studies different from the dB I'm seeing everywhere in spectograms etc?

Answer 1

The confusion arises from the fact that the decibel (dB) is a relative unit of measurement, not an absolute one. The dB scale in audio can reference different things depending on the context, which can make interpreting dB values somewhat challenging.

For example, the dB value in digital audio is usually a measure of amplitude relative to the maximum possible amplitude before clipping (distortion) occurs. This is called dBFS (decibels relative to Full Scale), where 0 dBFS is the maximum possible level, and everything else is negative. So, when you see a dB value of -40 or -10 in your Sonic Visualizer app, it's showing you how much quieter the signal is compared to the maximum possible level.

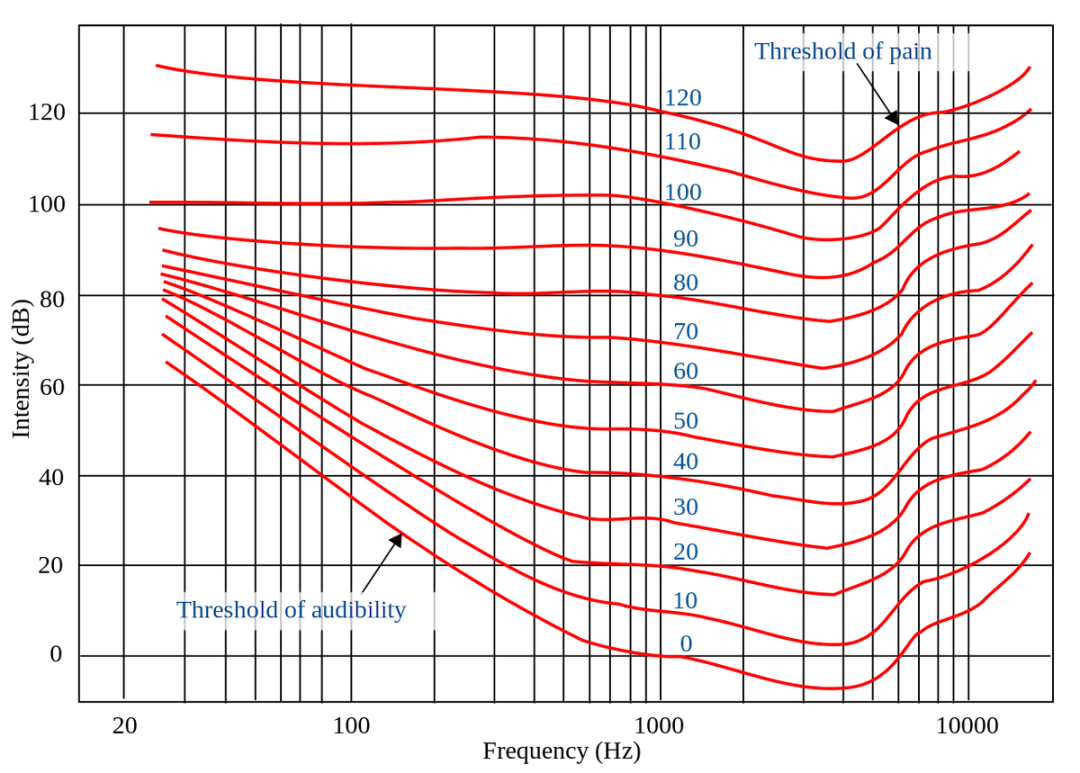
On the other hand, when we talk about the audibility threshold in terms of decibels, we're usually using a different reference point, often Sound Pressure Level (SPL). The "threshold of hearing" is typically defined as 0 dB SPL, which corresponds to the quietest sound that the average human ear can hear. Sounds that are louder than this threshold have positive dB SPL values, and sounds that are quieter have negative dB SPL values. However, in practice, sounds quieter than the threshold of hearing are not usually discussed, because they're... well, inaudible.

So, to directly answer your question: the dB values you're seeing in your audio software don't directly translate to dB SPL values in audibility studies. They're relative to different reference points. The 0 dBFS in digital audio doesn't correspond to 0 dB SPL. Instead, 0 dBFS is the highest level a digital system can handle, while 0 dB SPL is the quietest sound the average human ear can detect.

Moreover, the mapping from dBFS to dB SPL can vary widely depending on factors like the specific hardware used for playback, the volume setting on that hardware, distance from the speakers, ambient noise levels, and so on. There's not a fixed conversion between the two: what might be -20 dBFS in your digital audio file could end up being played back at any number of dB SPL in the real world, depending on these factors.

Answer 2

dB is a logarithmic metric against a well defined reference. There are many different types of "dB" depending on the nature of the signal. For example voltage measurements can be in dBv, dBu, or dBm depending on what reference is used.

What you are seeing in is dBDFS, (dB digital full scale), which is relative the largest number you represent without clipping. For example the power level of a full scale digital sine wave is -3dBDFS.

I read online that the human ear starts to hear near -10-10dB.

This is specifically dBSPL (sound pressure level), which is a measurement of the acoustic pressure at the human ear location relative to a reference pressure of $20 \mu Pa$. The hearing threshold is highly dependent on frequency so a better metric would be dBSPL(A) which applies a frequency weighing (the so -called "A-weighting") to the pressure.

It's very difficult to predict the sound pressure at the ear (in dBSPL(A) or similar) from the dBDFS level of the sound file. The signal passes through A LOT of different transformations before it reaches the ear. The most obvious one is a volume control but the are is also more postprocessing, D/A gain, amplifier gain, loudspeaker sensitivity and the transfer function from the loudspeaker to the ear. The latter one is extremely complicated, so the most common way of doing the conversion is to use a calibration procure.

Why are the dB reported in these audibility studies different from the dB I'm seeing everywhere in spectograms etc?

Because they are different "types" of dB and cannot easily be converted to each other without A LOT of extra information.

Answer 3

There's one broad standard that is only strictly followed in certain conditions.

The cinema standard is a 500-2000 Hz band-limited pink noise signal with digital RMS amplitude of -20 dB full scale will produce 85 dBC SPL slow response at the listening position. dBC SPL refers to both a perceptual weighing curve, a time function, and a reference pressure of 20 uPa. This is formalized by Dolby.

The easiest way to experience this is if you have a home theater AV receiver with an auto calibration mic. Do the calibration, then set the volume control to 0 dB, this should set the playback side for this standard. Next, view some movie content, e.g, from a Blu-Ray. Apple content also tends to use this relationship.

You'll notice that the volume of the content seems low. That's because most TV content is mixed at least 10 dB louder, i.e. -30 RMS dBFS produces 85 dBc. Radio and music content can easily be 15-20 dB louder than the cinema standard.

Other media, such as the BBC, ITU, YouTube, etc. have their own volume standards with their own perceptual models, time-response functions and standards.

Answer 4

I read online that the human ear starts to hear near -10-10dB.

The thing that bothers me is that this reference:

is used instead of this reference:

I've always thunked that the 0 dB curve is what defines 0 dB SPL at 1000 Hz.