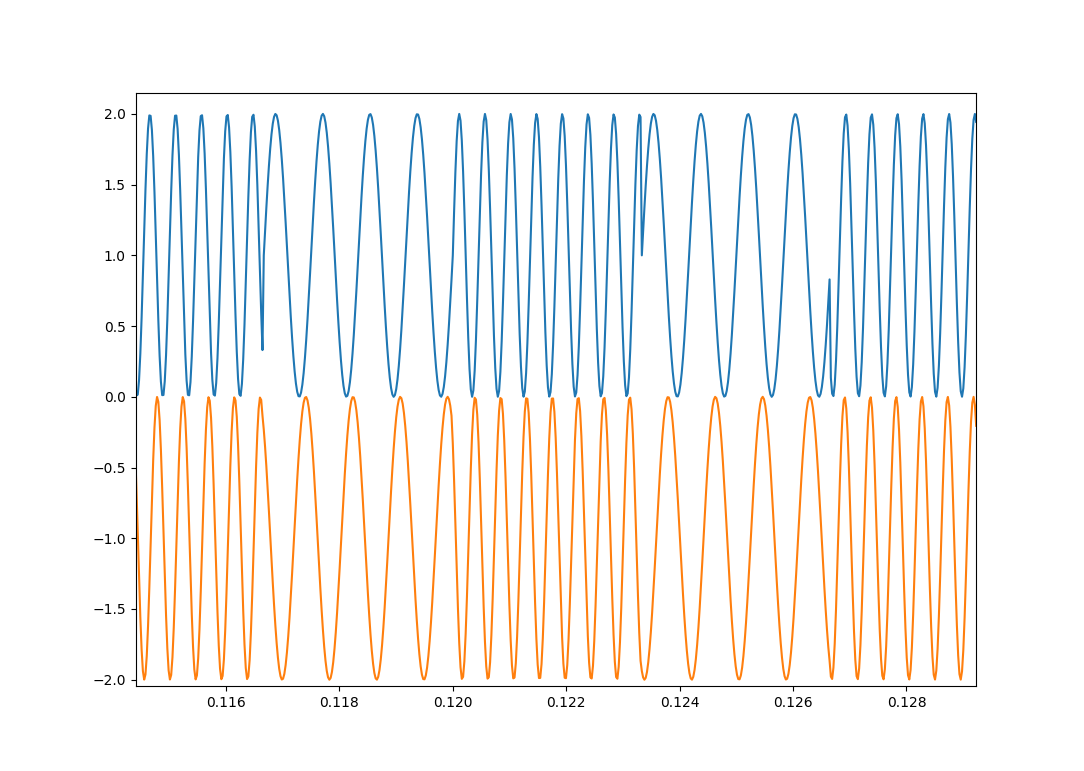
The phase of your output signal is not continuous, because you have implemented the phase as the output of one of two independent frequency oscillators both starting at time $0$, so their phases are not synchronized when you switch frequencies.
We can get continuous phase by noting that the phase is the integral of instantaneous frequency over time:
$$\phi(t) = \int^t 2\pi f_i(\tau) d\tau = \int^t d\phi(\tau)$$
or the discrete time equivalent, normalizing $1$ sample of a Nyquist frequency wave to be equivalent to $\pi$ radians of phase increment (or, equivalently, setting $\Delta t = 1/F_s$, the duration of $1$ sample time):
$$ \phi[n] = \sum_{k=0}^{n} \dfrac{\pi}{\frac{F_s}{2}} f_i[k] = \sum_{k=0}^{n} 2\pi f_i[k]\Delta t =\sum_{k=0}^{n} \Delta\phi[k]$$
Demonstrating this with a small addition to your script:
import numpy as np
import random
import matplotlib.pyplot as plt
sampling_rate = 44100
baud_rate = 300
samples_per_bit = 1.0 / baud_rate * sampling_rate
# tones representing bits, dummy data (0,1)
bits_in_tones = [1200, 2200] * 100
random.shuffle(bits_in_tones)
bit_arr = np.array(bits_in_tones)
symbols_freqs = np.repeat(bit_arr, samples_per_bit)
t = np.arange(0, len(symbols_freqs) / sampling_rate, 1.0 / sampling_rate)
signal = np.sin(2.0 * np.pi * symbols_freqs * (t))
plt.plot(signal)
plt.show()
# New lines here demonstrating continuous phase FSK (CPFSK)
delta_phi = symbols_freqs * np.pi / (sampling_rate / 2.0)
phi = np.cumsum(delta_phi)
signal2 = np.sin(phi)
plt.plot(t, signal+1.0)
plt.plot(t, signal2-1.0)
plt.show()
Of course this implementation runs into numerical problems: as we keep incrementing phase, we will begin to lose precision. Also, it is bad practice to pass arguments to the $\sin()$ function that are far outside of the interval $[-2\pi,2\pi]$. You'll want to wrap the phase to keep it within $[0,2\pi)$ as you cumulatively sum it, as opposed to the naive, convenient cumulative sum I've done here.
Here is a comparison of the output of your original switched FSK implementation vs. the CPFSK implementation: