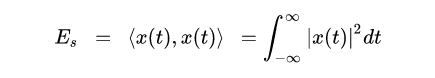2
$\begingroup$

What is energy in terms of signal processing? You can read here, that energy is

enter image description here

I just need a simple everyday-example for that energy. And yes, bringing an example like

$x(t)$ representing the potential of an electrical signal propagating across a transmission line

is still too complicated for me.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

Pick up an apple and raise it one meter. You have just spent (approximately) one joule of energy. You had to spend energy because the action (raising the apple) goes against a force (Earth's gravity).

The same is true for electrons. An electrical signal is nothing but electrons moving in an electric field. Causing these electrons to move takes energy, exactly like moving a train or an apple.

In the formula you posted, $x(t)$ is a voltage causing electrons to move in a resistance of $1\,\Omega$, and $E_s$ is the energy required to make those electrons move around in the resistor, causing a current to develop.

Improve this answer
$\endgroup$
4
  • $\begingroup$ But what if I am thinking about signal as general function of t. Moreover, your explanation will fail in discrete time case $\endgroup$
    – struct
    Commented May 11, 2018 at 5:48
  • $\begingroup$ The "energy" of an abstract signal that is not associated with a physical voltage or current is just an abstract "measure" of the signal. You can think of it as "what energy would this signal have if it were a voltage". As for discrete signals, I interpret their energy as "the energy this signal will have when converted to continuous time". $\endgroup$
    – MBaz
    Commented May 11, 2018 at 16:29
  • $\begingroup$ Can you provide mote details about "when converted to continuous time"? I suppose disctete time signals as pulses with zero time duration How one can get energy of such signals in physical meaning? $\endgroup$
    – struct
    Commented May 18, 2018 at 20:22
  • $\begingroup$ @JanFilip The way I think about it is that discrete-time signals do not exist in the physical world -- they are just sequences of numbers. However, one can sample a physical signal and convert it to discrete. Many of the signal's properties, including its energy, are inherited by the discrete signal. In the same way, when discrete signal is interpolated and converted to continuous time, the CT signal inherits the energy of the DT signal (up to a constant factor). Let me know if this explanation helps. $\endgroup$
    – MBaz
    Commented May 18, 2018 at 21:52
0
$\begingroup$

Let's understand why this definition is complicated to begin with.

Energy of signal is defined as $E_s := \langle x(t),x(t) \rangle$ which could equate to, in the continuous case as $ \Rightarrow \int |x(t)|^2 dx$ and in the discrete case as $ \Rightarrow \sum |x[n]|^2 $. Here, the energy is taken as an inner product $\langle , \rangle$ of the signal $x(t)$ on itself. An inner product, being a linear algebra concept, hints at the notion of "a signal as a vector". This little hint helps a ton with developing an understanding for spectral analysis or stochastic processes later in studies. Signals, as in spectral theory, could also be defined on complex values $(s=\alpha + i \omega)$. In such case, the formula above fails and must accommodate a conjugate as well $\int x(t) \overline{x(t)} dx$.

Further, it has been explicitly stated in signal-processing books (such as this ) that a signal is, generally, not (always) a physical concept. Thus, interpretation of the concept of "energy" in terms of signals could be misleading. It is considered best to comprehend "signal-energy" as "weight of a signal". Even in the most assumed case, where an EM wave acts as a signal; the definition of signal-energy doesn't match the exact semantic of energy as in physics.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.