Just to restate everything for clarity, the Compressed Sensing problem is defined as the following: given a signal $x$ of length $N$, we measure the projection of $x$ by some projection operator, $\Phi$ of size $M \times N$,
$$ y = \Phi x,$$
where $y$ is are our $M$ measurements of the original signal. We could also state this in terms of the inner products of $x$ with the rows of $\Phi$,
$$ y_i = \left< \phi_i, x\right>.$$
In the CS sampling procedure we have an inherent dimensionality reduction since we are taking a projection of $x$. The degree of this dimensionality reduction is usually referred to in terms of the ratio $\frac{M}{N}$ (aka the subsampling ratio or "Subrate"). By obtaining $y$ during acquisition we are simultaneously performing acquisition and dimensionality-reduction rather than full-resolution sampling followed by compression (DCT, DWT, etc.). The measurements $y$ are read off of the sensor, quantized (still an open problem, but decent results can be obtained through simple scalar quantization. See Laska's dissertation for more novel approaches), entropy coded (pick your flavor), and then transmitted or stored.
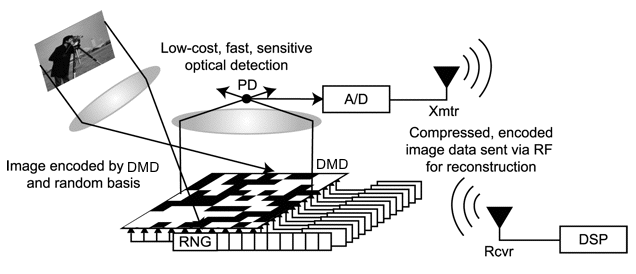
You touch on a good key point when it comes to CS signal acquisition, namely, if the calculation of this projection is costly then the advantage of CS for acquisition systems would appear small. However, depending on the context and type of signal, such projections can be accomplished in the analog domain (requiring no computation). The single-pixel camera (SPC) is a great example of this.
Researchers have also been able to greatly reduce sampling times for MRI using CS techniques. Specifically, conventional MRI techniques sample along different radial lines within the frequency domain. Each radial line is a measurement of the MRI device requiring some sampling time. Traditionally, for higher resolution MRI, more radial lines must be acquired, incurring longer acquisition time (problematic for the MRI of small children). However, this process can also be characterized as linear projections within the frequency domain. Because of this, CS techniques can be used to recover MRI images from many fewer measurements, allowing for high resolution MRI with much shorter MRI appointments. This was, in fact, the context in which CS was first applied. For more info, this paper by Lustig et al might be a good starting point.
In summation, the usefulness of CS to a particular context really depends on how you implement the projection. Getting it right can require some out-of-the-box thinking to come up with a novel sensing strategy. Thankfully, frameworks such as the SPC are generalizeable to a wide range of different signal contexts, so we don't have to re-invent the wheel every time.

