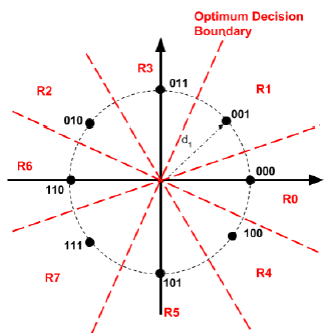
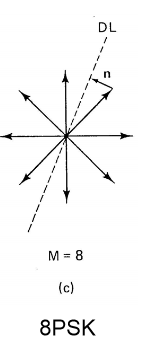
I'm trying to understand how to derive the BEP for 8PSK by geometrically looking at the decision regions.
Say I have this 8PSK signal set and the corresponding decision boundaries.
I assume that '000' is sent, and I want to calculate the BEP. So I have
$$P_b(e) = \frac{1}{3}(1 \times R_1 + 1 \times R_2 + 2 \times R_3 + 1 \times R_4 + 2 \times R_5 + 2 \times R_6 + 3 \times R_7)$$
Since $R_1 = R_4$, $R_3 = R_5$ and $R_2 = R_7$ by symmetry, the above can be simplified to
$$P_b(e) = \frac{2}{3}(R_1 + 2R_2 + 2R_3 + R_6)$$
Then we have
$$R_1 + R_2 + R_3 + R_6 = P\Big(n > d_1sin\frac{\pi}{8}\Big)\tag{1}$$$$R_1 + R_2 + R_3 + R_6 = P\Big(n > d_1\sin\frac{\pi}{8}\Big)\tag{1}$$
and
$$R_2 + R_3 = P\Big(n_0 > d_1sin\frac{3\pi}{8}\Big) \times P\Big(n_1 < d_1sin\frac{\pi}{8}\Big)\tag{2}$$$$R_2 + R_3 = P\Big(n_0 > d_1\sin\frac{3\pi}{8}\Big) \times P\Big(n_1 < d_1\sin\frac{\pi}{8}\Big)\tag{2}$$
I'm having a hard time visualizing and understanding how $(1)$ and $(2)$ are obtained.
I understand that $n$ is the noise vector, and $n_0$ and $n_1$ are the horizontal and vertical components of the noise vector, and we are trying to find out how far $n$ or $n_0$ and $n_1$ will go to "push" the signal into the wrong decision region.
I've seen the following from this website, and it seems to explain $(1)$, that is, the noise vector $n$ "pushes" the signal past the decision line (dl) into the wrong decision regions $R_1 + R_2 + R_3 + R_6$.
But what about $(2)$? I don't know why we have $sin\frac{3\pi}{8}$$\sin\frac{3\pi}{8}$ and $sin\frac{\pi}{8}$$\sin\frac\pi8$.
Also, is the noise vector always perpendicular to the decision boundaries?