This is a continuation of the discussion here. I would comment there, but I don't have 50 rep so I'm asking a new question.
Here's how I understand the DCT step in the MFCC calculation process: The rationale behind it is to separate the correlation in the log-spectral magnitudes (from the filterbank) due to the overlapping of the filters. Essentially, the DCT smooths the spectrum representation given by these log-spectral magnitudes.
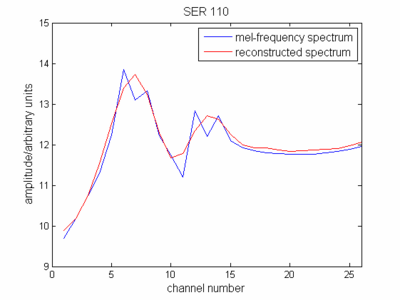
Would it be correct to say that the blue line in the image below represents the spectrum as represented by the vector of log-spectral magnitudes, and the red line is that vector once its been DCT-ified?