fs = 2^10;        %sample frequency in Hz
T  = 1/fs;        %sample period in s
L  = 2^20;        %signal length
t  = (0:L-1) * T; %time vector

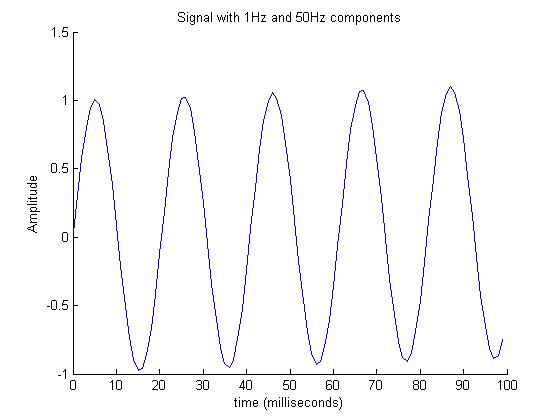
A1 = 0.2; %amplitude of x1 (first signal)
A2 = 1.0; %amplitude of x2 (second signal)
f1 = 1;   %frequency of x1
f2 = 50;  %frequency of x2

x1 = A1*sin(2*pi*f1 * t); %sinusoid 1
x2 = A2*sin(2*pi*f2 * t); %sinusoid 2
y  = x1 + x2;

%Plot signal
figure;
set(gcf,'Color','w'); %Make the figure background white
plot(fs*t(1:100), y(1:100));
set(gca,'Box','off'); %Axes on left and bottom only
str = sprintf('Signal with %dHz and %dHz components',f1,f2);
title(str);
xlabel('time (milliseconds)');
ylabel('Amplitude');

%Calculate spectrum
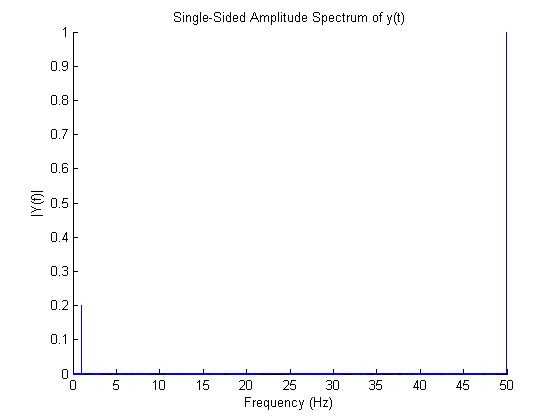
Y = fft(y)/L;
ampY = 2*abs(Y(1:L/2+1));
f = fs/2*linspace(0,1,L/2+1);
i = L/fs * (max(f1,f2)) + 1; %show only part of the spectrum

%Plot spectrum.
figure;
set(gcf,'Color','w');  %Make the figure background white
plot(f(1:i), ampY(1:i));
set(gca,'Box','off');  %Axes on left and bottom only
title('Single-Sided Amplitude Spectrum of y(t)');
xlabel('Frequency (Hz)');
ylabel('|Y(f)|');

fs = 2^10;        %sample frequency in Hz
T  = 1/fs;        %sample period in s
L  = 2^20;        %signal length
t  = (0:L-1) * T; %time vector

A1 = 0.2; %amplitude of x1 (first signal)
A2 = 1.0; %amplitude of x2 (second signal)
f1 = 1;   %frequency of x1
f2 = 50;  %frequency of x2

x1 = A1*sin(2*pi*f1 * t); %sinusoid 1
x2 = A2*sin(2*pi*f2 * t); %sinusoid 2
y  = x1 + x2;

%Plot signal
figure;
set(gcf,'Color','w'); %Make the figure background white
plot(fs*t(1:100), y(1:100));
set(gca,'Box','off'); %Axes on left and bottom only
str = sprintf('Signal with %dHz and %dHz components',f1,f2);
title(str);
xlabel('time (milliseconds)');
ylabel('Amplitude');

%Calculate spectrum
Y = fft(y)/L;
ampY = 2*abs(Y(1:L/2+1));
f = fs/2*linspace(0,1,L/2+1);
i = L/fs * (max(f1,f2)) + 1; %show only part of the spectrum

%Plot spectrum.
figure;
set(gcf,'Color','w');  %Make the figure background white
plot(f(1:i), ampY(1:i));
set(gca,'Box','off');  %Axes on left and bottom only
title('Single-Sided Amplitude Spectrum of y(t)');
xlabel('Frequency (Hz)');
ylabel('|Y(f)|');

A graph of a fft (Fast Fourier Transform) allows us to visualize different frequencies. This example is adapted from Matlab's fft help. The following figure illustrates a time signal with two frequencies. Note how it is difficult to see the 1Hz component in the time domain.

fs = 2^10;        %sample frequency in Hz
T  = 1/fs;        %sample period in s
L  = 2^20;        %signal length
t  = (0:L-1) * T; %time vector

A1 = 0.2; %amplitude of x1 (first signal)
A2 = 1.0; %amplitude of x2 (second signal)
f1 = 1;   %frequency of x1
f2 = 50;  %frequency of x2

x1 = A1*sin(2*pi*f1 * t); %sinusoid 1
x2 = A2*sin(2*pi*f2 * t); %sinusoid 2
y  = x1 + x2;

%Plot signal
figure;
set(gcf,'Color','w'); %Make the figure background white
plot(fs*t(1:100), y(1:100));
set(gca,'Box','off'); %Axes on left and bottom only
str = sprintf('Signal with %dHz and %dHz components',f1,f2);
title(str);
xlabel('time (milliseconds)');
ylabel('Amplitude');

%Calculate spectrum
Y = fft(y)/L;
ampY = 2*abs(Y(1:L/2+1));
f = fs/2*linspace(0,1,L/2+1);
i = L/fs * (max(f1,f2)) + 1; %show only part of the spectrum

%Plot spectrum.
figure;
set(gcf,'Color','w');  %Make the figure background white
plot(f(1:i), ampY(1:i));
set(gca,'Box','off');  %Axes on left and bottom only
title('Single-Sided Amplitude Spectrum of y(t)');
xlabel('Frequency (Hz)');
ylabel('|Y(f)|');

A graph of a fft (Fast Fourier Transform) allows us to visualize different frequencies. This example is adapted from Matlab's fft help. The following figure shows the first 100 out of $2^{20}$ samples of a time signal with two frequencies. Note how it is difficult to see the 1Hz component in this figure.

fs = 2^10;        %sample frequency in Hz
T  = 1/fs;        %sample period in s
L  = 2^20;        %signal length
t  = (0:L-1) * T; %time vector

A1 = 0.2; %amplitude of x1 (first signal)
A2 = 1.0; %amplitude of x2 (second signal)
f1 = 1;   %frequency of x1
f2 = 50;  %frequency of x2

x1 = A1*sin(2*pi*f1 * t); %sinusoid 1
x2 = A2*sin(2*pi*f2 * t); %sinusoid 2
y  = x1 + x2;

%Plot signal
figure;
set(gcf,'Color','w'); %Make the figure background white
plot(fs*t(1:100), y(1:100));
set(gca,'Box','off'); %Axes on left and bottom only
str = sprintf('Signal with %dHz and %dHz components',f1,f2);
title(str);
xlabel('time (milliseconds)');
ylabel('Amplitude');

%Calculate spectrum
Y = fft(y)/L;
ampY = 2*abs(Y(1:L/2+1));
f = fs/2*linspace(0,1,L/2+1);
i = L/fs * (max(f1,f2)) + 1; %show only part of the spectrum

%Plot spectrum.
figure;
set(gcf,'Color','w');  %Make the figure background white
plot(f(1:i), ampY(1:i));
set(gca,'Box','off');  %Axes on left and bottom only
title('Single-Sided Amplitude Spectrum of y(t)');
xlabel('Frequency (Hz)');
ylabel('|Y(f)|');