Excerpted from Jae S.Lim 2D signal and image processing ch.1, as an example of $2$-D circularly symmetric lowpass filter with a cutoff frequency of $\omega_c$ radians per sample, whose impulse response is given by: $$h[n_1,n_2] = \frac{\omega_c}{2\pi \sqrt{n_1^2 + n_2^2} } J_1 \big( \omega_c \sqrt{n_1^2 + n_2^2} \big) $$
where $J_1$ is the Bessel function of the first kind and the first order...
Interested readers may consult the book for a derivation which is not untitive but nevertheless tractable; familiarity with Bessel functions is required but also provided as is.
At $n_1 = n_2 = 0$ the limiting value must be used:
$$h[0, 0] = \frac{\omega_c^2}{4\pi}$$
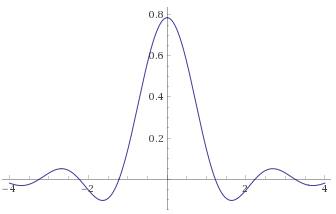
A slice through the middle of $h[n_1,n_2]$ with $\omega_c = \pi$: