1
$\begingroup$

A baseband signal is just a signal that has not been used to modify a carrier signal. Now, I have noticed that all baseband signals are low-pass too. Why is that?

I can understand why digital baseband signals are always low-pass: because the frequency 0 is always one of the constituent frequencies of a digital signal.

However, why must it always be the case for analog signals? I think that whether a signal modulates a carrier signal has nothing to do with the lower interval of its bandwidth. Why can't we have a baseband signal that is naturally bandpass: that is, why can't a device generate a (baseband) signal that is naturally bandpass (being bandpass without any filter)?

Could someone please explain this?

Edit:

Low-pass signal/channel: A channel/signal whose bandwidth starts from 0Hz.

enter image description here

Band-pass signal/channel: A channel/signal whose bandwidth does not start from 0Hz.

enter image description here

Improve this question
$\endgroup$

3 Answers 3

Reset to default
1
$\begingroup$

All signals have a bandwidth.

For example audio signals can be said to have a bandwwidth from 20 Hz to 20 kHz.

Now a telephone system had bandwidth from 300 Hz to 3400 Hz.

Some signals have bandwidth down to DC, but generally you don't want to include static air pressure as DC offset when recording audio.

Basically they all look like low pass systems because they all go near DC.

But for example a morse code transmitter sending a 1 kHz tone or nothing wilh have a narrow bandwidth around 1 kHz and no DC.

There are also digital coding systems that for infinite runs of signal don't have any DC, such as bipolar Manchester coding.

Improve this answer
$\endgroup$
5
  • $\begingroup$ "But for example a morse code transmitter sending a 1 kHz tone or nothing wilh have a narrow bandwidth around 1 kHz and no DC." Do you mean "between around 1kHz and DC (0Hz)"? When the simple sine signal of 1KHz is sent, then the frequency is clearly 1KHz. Then, when nothing is sent, the frequency is 0Hz. So, the bandwidth range will be from 0Hz (DC) to 1KHz. $\endgroup$
    – tryingtobeastoic
    Commented Dec 6, 2023 at 5:22
  • $\begingroup$ @tryingtobeastoic By your logic, if nothing is sent at, you have a bandwidth from 0 to infinity sending nothing. But if you imagine a speakes either transmitting 1kHz or nothing, it only requires the speaker or air or your ear to support 1 kHz, it does not matter if frequencies from 0 to 900 and from 1100 to infinity are not supported by the speaker, air, or your ears, because only the transmitted signal energy at 1khz matters. You could design the speaker with a bandpass of 1 kHz and nothing will change when transmitting 1 kHz or nothing. But it can't send 2kHz then. $\endgroup$
    – Justme
    Commented Dec 6, 2023 at 7:59
  • $\begingroup$ Consider this (possible) definition of the bandwidth required to transmit: a system has bandwidth B (from f1 to f2) if any extraneous signals on B would interfere with its communications. In this sense, the Morse code example might be said to have a bandwidth of 1 kHz: from 0 to 1000. $\endgroup$
    – MBaz
    Commented Dec 6, 2023 at 15:41
  • $\begingroup$ @MBaz If you used the signal to say modulate an FM carrier, any DC offset in the baseband 1 kHz beeping Morse code signal would bias the FM carrier too. If there is a DC offset among the 1 kHz beeping tone sent to speaker, it will bias the speaker cone from the midpoint. And speakers are not intended to handle DC. So for the 1 kHz beeping morse code signal to speaker, any DC will interfere the transmission so it must be ruled out from the signal bandwidth. Same rule about DC applies to e.g. Ethernet communication. $\endgroup$
    – Justme
    Commented Dec 6, 2023 at 16:05
  • $\begingroup$ @Justme I agree, and it seems to me you're basically saying the same thing I did: what happens at DC has an effect on the system's behavior, so it must be taken into account. On the other hand, a receiver with a BPF at its input is (ideally) not affected by signals outside its bandpass, so those do not need to be considered. $\endgroup$
    – MBaz
    Commented Dec 9, 2023 at 15:22
2
$\begingroup$

If "low-pass" is defined as "does not modulate a carrier", then most signals in nature are low-pass -- asking "why" is a bit like asking why trees have roots: nature simply didn't create/evolve signaling systems that are modulated.

However, if "low-pass" is defined as "its lowest frequency is 0", then there are many signals that are not low-pass. An example is visible light (interpreted as a wave): it is very high frequency and narrowband. Even sound does not reach 0 Hz.

By the way, you say that "digital baseband signals are always low-pass because the frequency 0 is always one of the constituent frequencies of a digital signal." This is not true: there are digital signals whose spectrum does not include zero, such as alternate mark inversion.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Do you happen to have a diagram of the frequency domain of bipolar AMI. If I directly see that the 0Hz frequency is not in the spectrum, that would be good. $\endgroup$
    – tryingtobeastoic
    Commented Dec 6, 2023 at 5:17
  • 1
    $\begingroup$ Slide 27 here: web.stanford.edu/class/ee179/lectures/notes14.pdf $\endgroup$
    – MBaz
    Commented Dec 6, 2023 at 15:43
  • $\begingroup$ Thank you so much :-) $\endgroup$
    – tryingtobeastoic
    Commented Dec 7, 2023 at 9:00
0
$\begingroup$

While understandable how one may get there, this definition does not make sense:

Band-pass signal/channel: A channel/signal whose bandwidth does not start from 0Hz.

While this may be true, it does not capture the real essence of band-pass. Thus, the definition of low-pass is similarly misguided.

Zero hertz is a degenerate case, because the concept of frequency is based on multiplicativity (division, technically, but that's just the inverse-multiplication function) and zero has a special meaning in multiplication. Any channel can exhibit a zero hertz signal, analog or digital, if you simply switch it off.

It would be better if you thought of all signals as having frequencies that are:

  • non-zero, so perhaps infinitesimally low-frequency, but greater than zero, and,
  • relative, such that one signal is of a lower, equal or higher frequency than another.

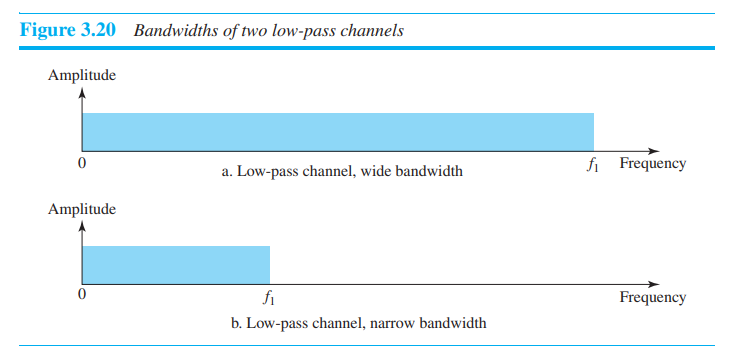
An ideal low-pass or high-pass filter would have a single cutoff. In the case of a low-pass, signals of frequencies lower than the cutoff are passed. Thus, low- (frequency) pass (not cut off but allowed to pass).

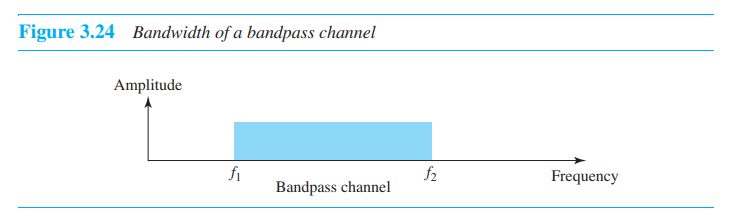
A band-pass filter passes a band, higher than a certain cutoff and lower than another cutoff. The frequency range between the cutoffs, that is passed, is the pass band, hence again the name.

There are many varieties of modulation but many useful ones have a net spectral effect of raising the frequency. A very common real-world use case is to modulate a low-frequency signal like human voice onto a high-frequency carrier like easily transmittable RF, or block coding for error correction.

Therefore very often a baseband signal will have a lower frequency than the desired channel frequency. Since the baseband is generally available on the transmission end of a channel and it is lower frequency, placing a low-pass filter between baseband input and modulation with a cutoff between the expected channel frequencies can often have the net effect of increasing the SNR.

As stated in some other answers, there are cases where it is useful to high-pass-filter your baseband as well. In some of these cases and depending on your application, it may be useful to band-pass-filter this baseband before modulation.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.