What is the phase of $Y(\omega)$ in relation to the phase of $X(\omega)$ where $x(t)$ modulated with an exponential carrier that has no phase shift?

Question

A homework problem of a free online course I am taking, asked to draw the magnitude and phase of $Y(\omega)$ where $y(t) = x(t) c(t)$ where $c(t)$ is $e^{j 3 \omega_{c} t}$ and where $X(\omega)$ is:

Simple phase modulation problem with a pretty simple exponential carrier.

Doing the Fourier Transform of the exponential carrier I got that it was an impulse of height $2 \pi$ at $\omega = 3 \omega_{c}$

Modulation (multiplication) equation in the frequency domain turns into $\frac{1}{2 \pi} X \left( \omega \right) * C \left( \omega \right)$ where $*$ is convolution.

(The $2 \pi$ cancels out the height of the impulse.) A frequency domain signal convolved with an impulse is just the signal moved over. So, my $\vert Y \left( \omega \right) \vert$ was just the $\vert X \left( \omega \right) \vert$ in the picture moved over where it centres at $3\omega_{c}$ like in the next picture.

For phase I broke up the equation into $Y \left( \omega \right) = \frac{1}{2 \pi} \left( 2 \pi e^{j0} \right) * \left( \vert X \left( \omega \right) \vert e^{j \angle X \left( \omega \right)} \right)$ because the carrier transform $C \left( \omega \right)$ has a zero phase.

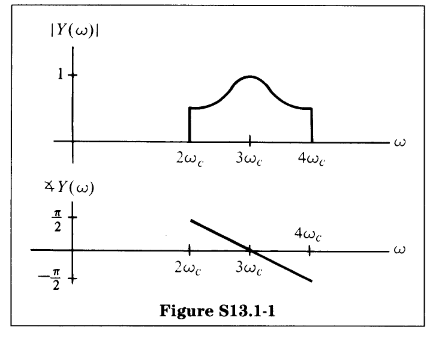
The homework solutions showed the following as the right answer for $Y \left( \omega \right)$ magnitude and phase, and I'm not sure why the phase is shifted. My intuition wants to tell me that the phase will match that of the original input signal because the only other phase in the equation is zero, so I would have thought the phase of $Y \left( \omega \right)$ would be the same as of $X \left( \omega \right)$.

I understand the rule about convolving with an impulse shifting the signal, but I guess I don't understand how it would influence the phase. Does that rule apply to just the magnitude or the full signal including the phase? What about convolution does that for the phase?

Answer 1

Does that rule apply to just the magnitude or the full signal including the phase?

The rules applies for the entire signal (magnitude, phase, real part, imaginary part, phase delay, group delay, level, etc.)

Does convolution do that for the phase?

Yes.

It's simply the shift property of the Fourier Transform. If $x(t) \leftrightarrow X(\omega)$ then

$$x(t) \cdot e^{j\omega_0 t} \leftrightarrow X(\omega-\omega_0)$$

It also follows directly from the convolution

$$f(t)*\delta(t-t_0) = f(t-t_0)$$