1
$\begingroup$

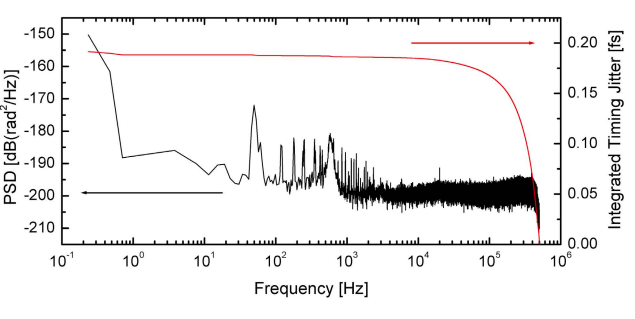
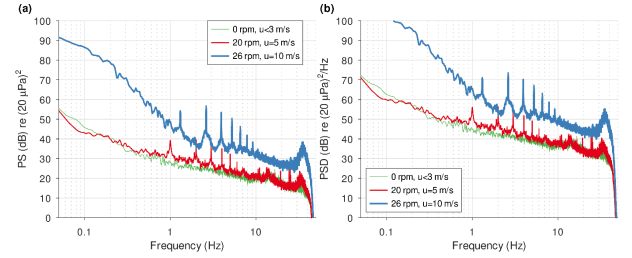
enter image description here enter image description here

In these power spectral density plots shown above (taken from the web 1,[2]), the spectra show a roll-off at the highest frequencies, as shown in the figure. For my own example, when I calculate PSD from time domain data taken with a sampling rate of 10 MSa/s using Welche's method, this roll-off appears at half the sampling rate in the spectra, which is after 5 MHz. Is this an artifact of the FFT or Welche's method, or could you please explain the reason behind this roll-off?

I have attached two figures where I see this roll-off. Thank you for your time.

Improve this question
$\endgroup$
1
  • 2
    $\begingroup$ It's just an indication that the dataset has a lowpass characteristic; that is, as frequency increases, the spectral content decays toward zero. This is a common attribute of signals, especially signals that have had anti-aliasing filtering applied before digitization. It doesn't have anything to do with Welch's method; for example, try generating white Gaussian noise and see that the PSD estimate is nearly flat. $\endgroup$
    – Jason R
    Commented Oct 18, 2022 at 15:02

1 Answer 1

Reset to default
2
$\begingroup$

It many cases this is caused by the data acquisition system. Most A/D converters have an anti-aliasing low pass filter. The choice of filter depends on the specific application but typically the cutoff frequency is at 80%-90% of the Nyquist frequency.

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ That is interesting! $\endgroup$
    – TM90
    Commented Oct 18, 2022 at 18:11
  • $\begingroup$ Thank you so much for the informed answer @Hilmar. Let me understand it more clearly. I understand that the DAQ may have anti-aliasing filters ranging close to the Nyquist frequency. Are these used in addition to the downsampling filters, (FIR filter)? $\endgroup$
    – Greta
    Commented Oct 19, 2022 at 5:55
  • 1
    $\begingroup$ It's the Analog to Digital converter that needs and anti-aliasing filter. Whether you need one for a D/A converter depends on the application. Down sampling also requires and anti-aliasing filter $\endgroup$
    – Hilmar
    Commented Oct 19, 2022 at 16:12
  • $\begingroup$ Thanks a lot, I got it. @Hilmar $\endgroup$
    – Greta
    Commented Oct 20, 2022 at 4:45

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.