Is scattering equivariant to multiplicative time warps? Defined as
$$ x(t) \rightarrow x(\tau(t)t),\ \text{sup}|\tau'(t)| < 1 $$
This post claims it holds approximately - what are the arguments, and approximation conditions?
Is scattering equivariant to multiplicative time warps? Defined as
$$ x(t) \rightarrow x(\tau(t)t),\ \text{sup}|\tau'(t)| < 1 $$
This post claims it holds approximately - what are the arguments, and approximation conditions?
The argument is simple: CWT center frequencies are distributed exponentially. Adjacent coefficients are hence related multiplicatively in frequency: the very wavelets are multiplicatively warped. The only mismatch is that of temporal support, which also varies exponentially.
For exact equivariance we require
$$ \text{supp}(x \star \psi_{\xi}) = \text{supp}(x \star \psi_{m\xi}), \ \forall m \in \mathbb{R} \tag{1} $$
where $\text{supp}=$ temporal support, and $\xi$ is some center frequency. This won't happen, as it's equivalent to fixing all wavelets' supports. Suppose a windowed signal with time support of 2, and wavelet $\psi_\xi$ of 10, and second wavelet $\psi_{2\xi}$:
supp(conv(x, psi_xi )) = 2 + 10 = 12supp(conv(x, psi_2xi)) = 2 + 10*2 = 22Note, if $\text{supp}(x) \ggg \text{supp}(\psi)$, then $(1)$ holds approximately:
supp(conv(x, psi_xi )) = 1000 + 10 = 1010supp(conv(x, psi_2xi)) = 1000 + 10*2 = 1020Alternatively, $\text{supp}(\psi_{\xi_0}) \approx \text{supp}(\psi_{\xi_1})$:
supp(conv(x, psi_xi )) = 1000 + 10 = 1010supp(conv(x, psi_2xi)) = 1000 + 10*2 = 1020"Commutativity" is what we're really showing; the result is:
$$ \text{warp}(\text{logfreq_shift}(x)) \Leftrightarrow \text{logfreq_shift}(\text{warp}(x)) $$
It's "equivariance" in the sense of, warping has same effect on transform of a signal regardless of signal's location on time-frequency plane (the "time" part guaranteed by translation equivariance).
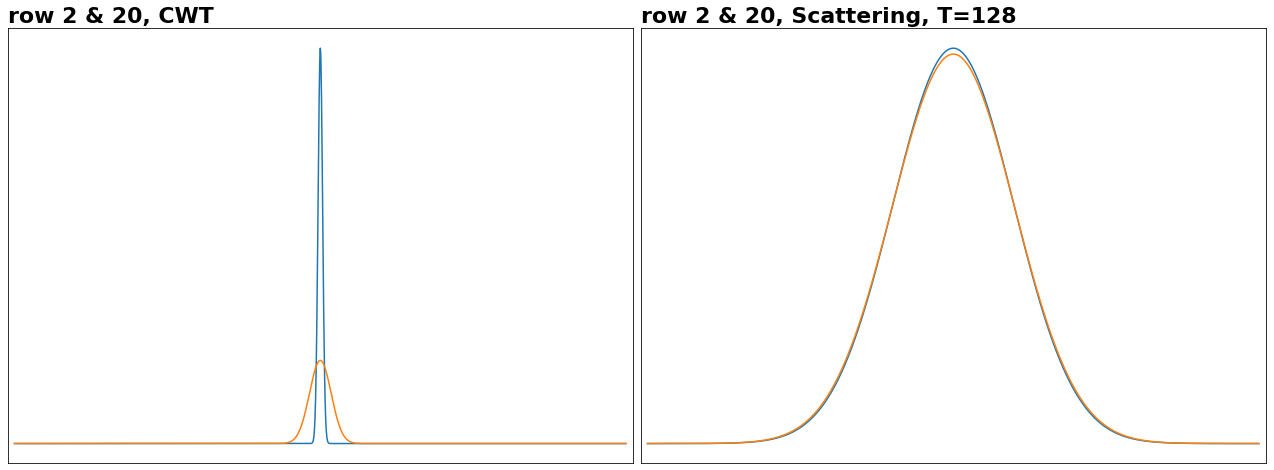
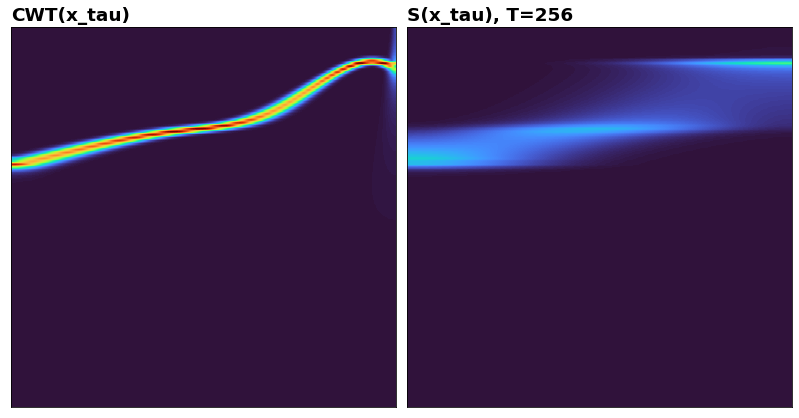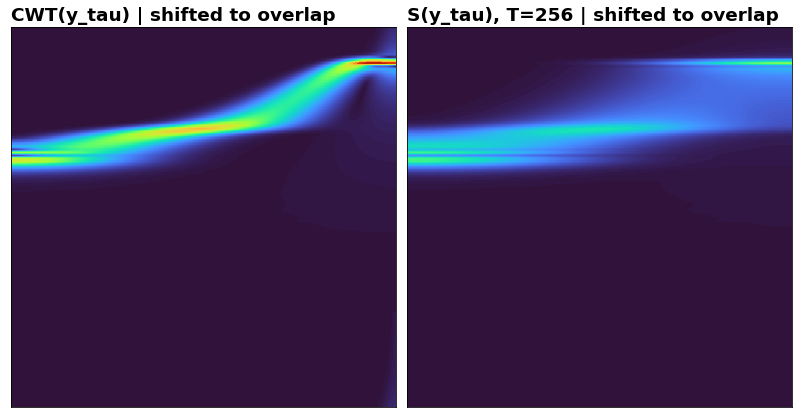
Last $\text{supp}$ result is key: scattering adds $T$ to effective support of every wavelet. Comparing impulse response${}^1$ of CWT and scattering, latter for all $T$; peak amplitude of each row has been normalized to be same for visual clarity (hence CWT', S'):

(1: unlike usual, scattering's IR can't be used as surrogate in convolution (i.e. overlap-adding weighted IRs) due to modulus nonlinearity)
Comparing distant rows from CWT & scattering:
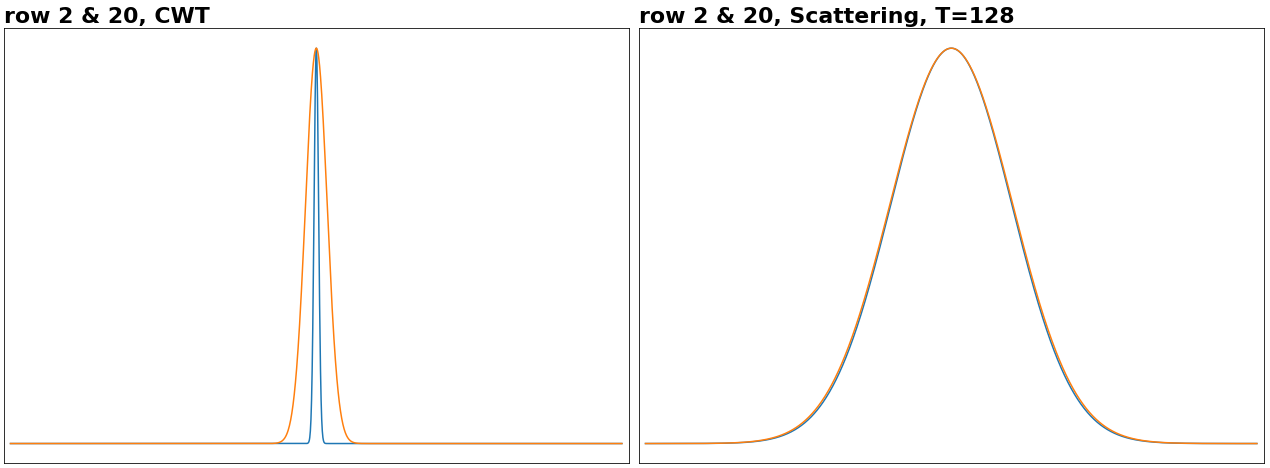
$x$ is swept from $128$ to $512\ \text{Hz}$, $y$ from $32$ to $128\ \text{Hz}$, both warped with same $|\tau(t)|$. Instantaneous ridges:
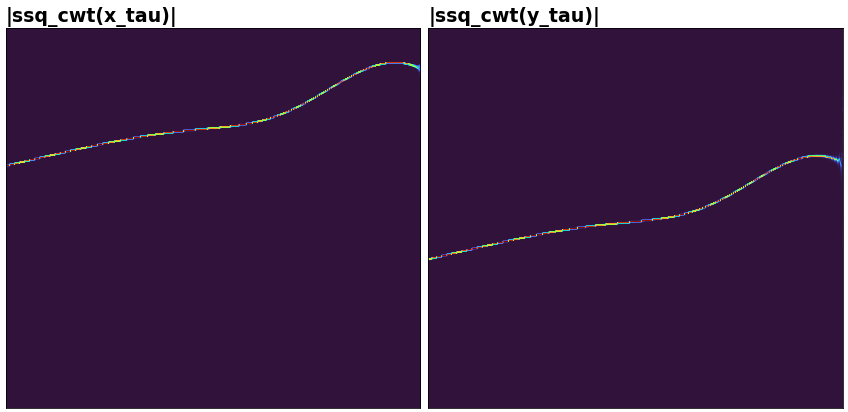
(Confirms the frequency ridges are exactly equivariant). CWT vs scattering:
There's commutativity in another sense: warping the signal can be traded for warping the wavelet, within a warp. Result from Lostanlen, sect 2.2.2):
Define "warp" as $\mathcal{W}_{(a,b)}x(t) = a x(at + b)$. Then,
$$ \begin{align} (\mathcal{W}_{(a,b)}x \star \psi)(t) &= \int_{-\infty}^{\infty} x(t')\psi\left(t - \frac{t' - b}{a}\right) dt' \\ &= a\left(x \star \mathcal{W}_{(1/a, 0)}\psi) \vphantom{\frac{.}{.}} \right)(at + b) \\ &= \mathcal{W}_{(a, b)} \left(x \star \mathcal{W}_{(1/a, 0)}\psi \vphantom{\frac{.}{.}} \right)(t) \\ \end{align} $$
Verbally: filtering warped signal == warping warp-filtered signal. Illustratively,
Verifying temporal supports:
2 * 3 + 5 = 112 * (3 + 5/2) = 2 * 5.5 = 11An equivalent verdict is much simpler - scale equivariance: scaling the input (in amplitude and time, i.e. "zooming"/"stretching") corresponds to scaling the output${}^2$. Hence, to state an equality, we undo this scaling with the inverse warp. It's a central motivation of wavelets - a "multi-scale zoom" (if we literally zoom on an image of a wavelet, it becomes another wavelet, scaled by the zoom factor). For scattering, this only holds above the scale of time-shift invariance, $T$ (see 'impulse response').
2: more precisely, corresponds to transforming the representation into the scaled region of the time-frequency plane. So a temporal stretching, and a log-frequency shift, such that new peak "resonant" wavelets are original ones scaled.
With steps:
$$ \begin{align} (\mathcal{W}_{(a,b)}x \star \psi)(t) &= \int_{-\infty}^{\infty} a x(au + b)\psi(t - u) du \\ t' = au + b &\Rightarrow u = (t' - b)/a,\ dt'=adu \\ &\Rightarrow \int_{-\infty}^{\infty} x(t')\psi\left(t - \frac{t' - b}{a}\right) dt' \\ \end{align} $$
$$ \begin{align} \left(x \star \mathcal{W}_{(1/a,0)}\psi \vphantom{\frac{.}{.}} \right)(t) & = \int_{-\infty}^{\infty} x(t - u) \frac{1}{a}\psi (u/a)du \\ t' = t - u \Rightarrow u = t - t',&\ dt' = -du,\ \infty \rightarrow -\infty \\ & \Rightarrow \frac{1}{a}\int_{-\infty}^{\infty} x(t') \psi ((t-t')/a)dt' \\ \mathcal{W}_{(a, b)}: t &\rightarrow at + b,\ \times a \\ & \Rightarrow \int_{-\infty}^{\infty} x(t') \psi ((at + b -t')/a)dt' \\ & = \int_{-\infty}^{\infty} x(t') \psi \left(t - \frac{t' - b}{a}\right)dt' \\ \end{align} $$
concludes the proof.
Scattering's contribution is more significant when realizing that CWT's scale equivariance includes amplitude scaling: $\mathcal{W}_{(a,b)}x(t) = ax(at + b)$. Scattering equalizes these as well; raw coefficients: