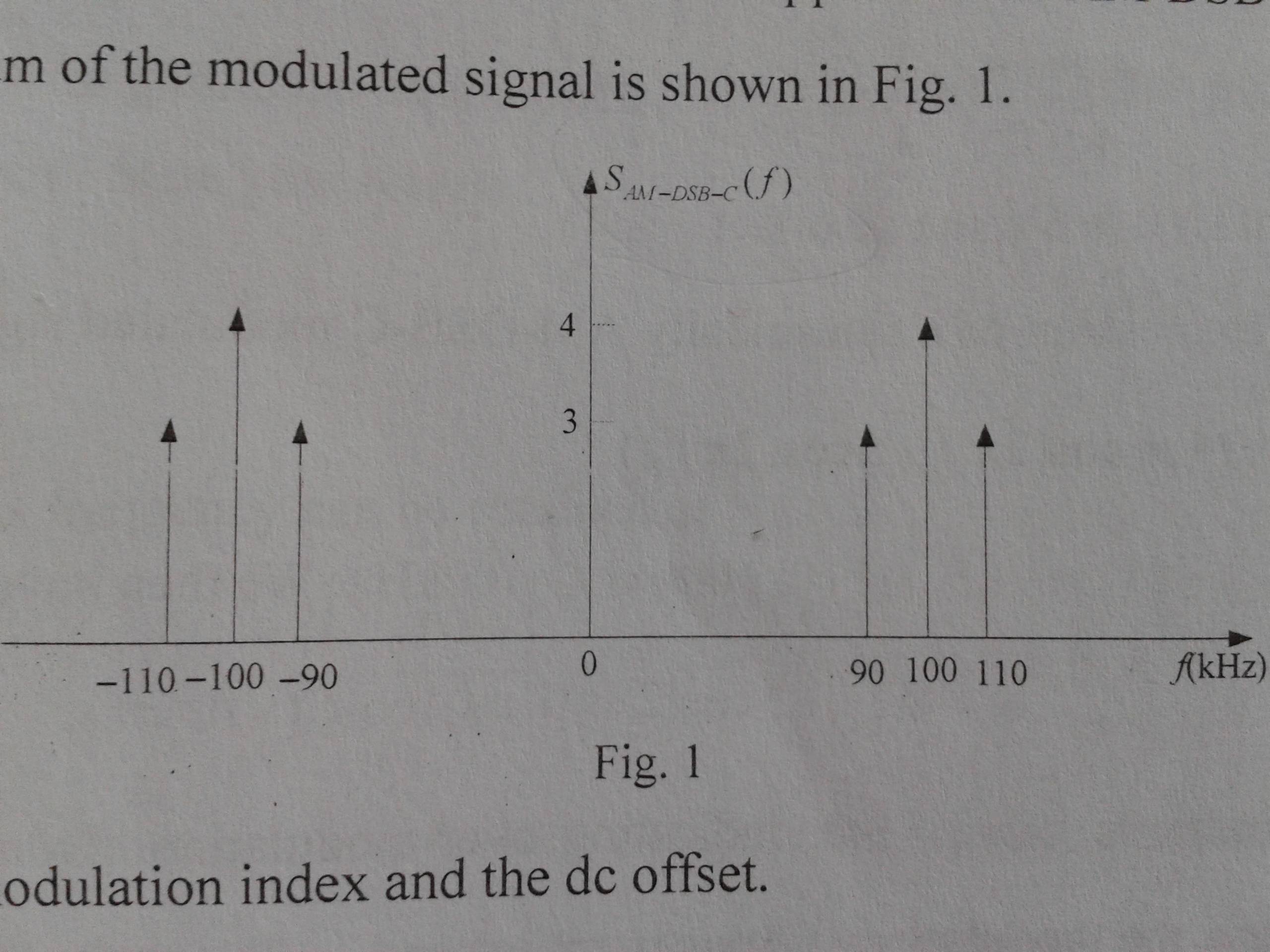
I have a question form my teachers, and I cannot understand why I can find out the modulation index form the figure.
The question provide a Figure like this:
And the information signal is a sinusoidal test signal with peak amplitude $6\ \rm V$ and is applied to an AM-DSB-C modulator, the Fourier spectrum of the modulated signal is shown above.
The solution is like this:
As $\displaystyle \frac {\frac {Acm}{4}}{\frac{Ac}{2}}=\frac{3}{4}$, $m=1.5$, so the modulation index is $1.5$ Moreover as $\displaystyle m=\frac{x}{c}$, the peak amplitude of $s(t)$ is $6\ \rm V$, so the DC offset($c$) is $4\ \rm V$.
I know where is the $\displaystyle \frac {Ac}{2}=4$ come form
as $s_{AM-DSB-C}(t)=A\big(s(t)+c\big)\cos(2 \pi f_c t)$
\begin{align} \mathcal F\left\{s_{AM-DSB-C}(t)\right\} &=S_{AM-DSB-C}(f)\\ &=\frac{A}{2}\big[S(f-f_c)+S(f+f_c)\big] + \frac {Ac}{2} \big[\delta (f-f_c) + \delta (f+f_c)\big] \end{align}
so, form the second term we can get $\displaystyle\frac {Ac}{2}=4$
But, I cannot understand how the solution can get $\displaystyle \frac {Acm}{4}=3$.